Multidimensional orthogonal matching pursuit: theory and application to high accuracy joint localization and communication at mmWave
Paper and Code
Aug 24, 2022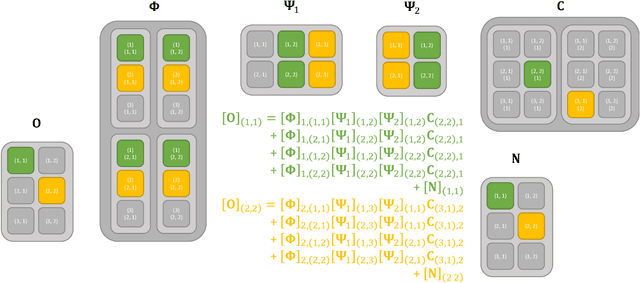
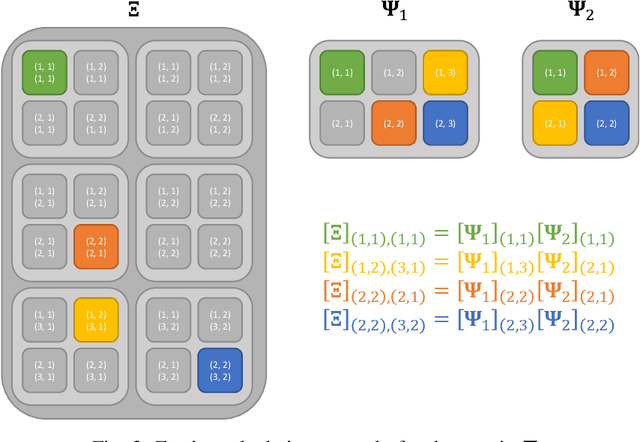
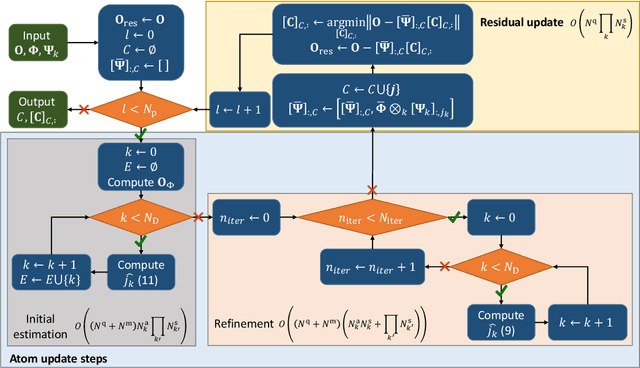
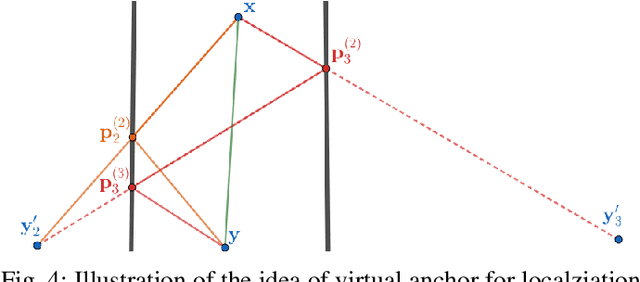
Greedy approaches in general, and orthogonal matching pursuit in particular, are the most commonly used sparse recovery techniques in a wide range of applications. The complexity of these approaches is highly dependent on the size of the dictionary chosen to represent the sparse signal. When the dictionary has to be large to enable high accuracy reconstructions, greedy strategies might however incur in prohibitive complexity. In this paper, we propose first the formulation of a new type of sparse recovery problems where the sparse signal is represented by a set of independent and smaller dictionaries instead of a large single one. Then, we derive a low complexity multdimensional orthogonal matching pursuit (MOMP) strategy for sparse recovery with a multdimensional dictionary. The projection step is performed iteratively on every dimension of the dictionary while fixing all other dimensions to achieve high accuracy estimation at a reasonable complexity. Finally, we formulate the problem of high resolution time domain channel estimation at millimeter wave (mmWave) frequencies as a multidimensional sparse recovery problem that can be solved with MOMP. The channel estimates are later transformed into high accuracy user position estimates exploiting a new localization algorithm that leverages the particular geometry of indoor channels. Simulation results show the effectiveness of MOMP for high accuracy localization at millimeter wave frequencies when operating in realistic 3D scenarios, with practical MIMO architectures feasible at mmWave, and without resorting to perfect synchronization assumptions that simplify the problem.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge