Multi-fidelity power flow solver
Paper and Code
May 26, 2022
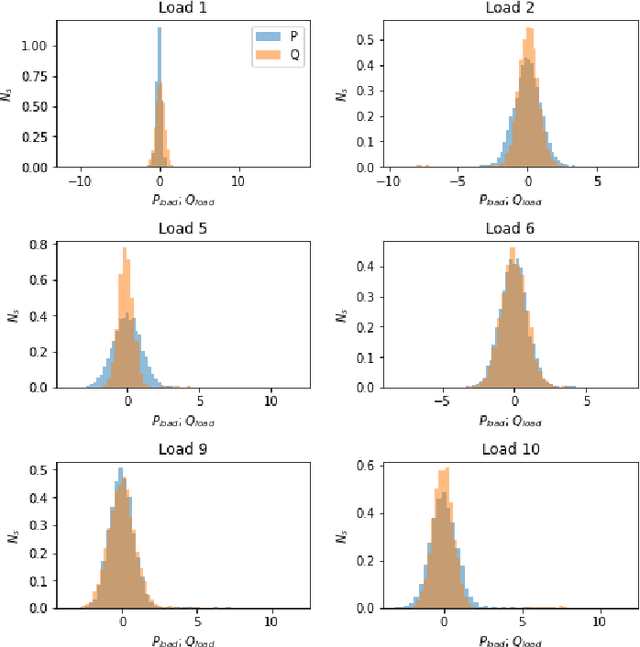
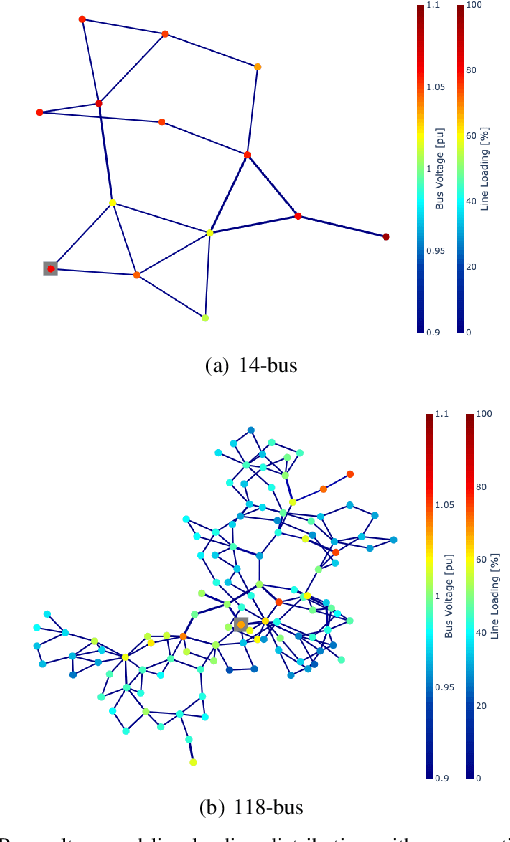
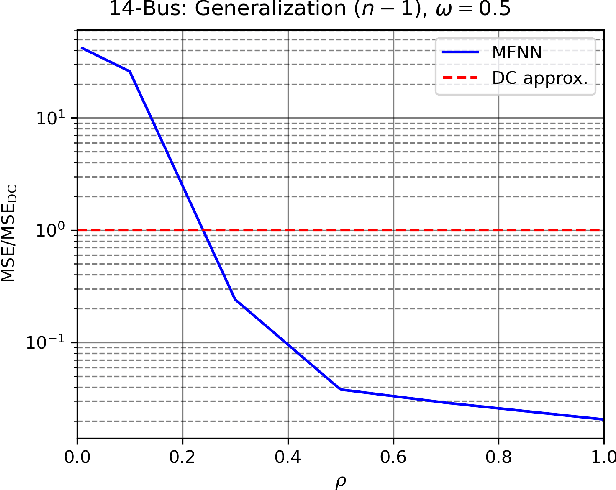
We propose a multi-fidelity neural network (MFNN) tailored for rapid high-dimensional grid power flow simulations and contingency analysis with scarce high-fidelity contingency data. The proposed model comprises two networks -- the first one trained on DC approximation as low-fidelity data and coupled to a high-fidelity neural net trained on both low- and high-fidelity power flow data. Each network features a latent module which parametrizes the model by a discrete grid topology vector for generalization (e.g., $n$ power lines with $k$ disconnections or contingencies, if any), and the targeted high-fidelity output is a weighted sum of linear and nonlinear functions. We tested the model on 14- and 118-bus test cases and evaluated its performance based on the $n-k$ power flow prediction accuracy with respect to imbalanced contingency data and high-to-low-fidelity sample ratio. The results presented herein demonstrate MFNN's potential and its limits with up to two orders of magnitude faster and more accurate power flow solutions than DC approximation.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge