Modeling natural language emergence with integral transform theory and reinforcement learning
Paper and Code
Nov 30, 2018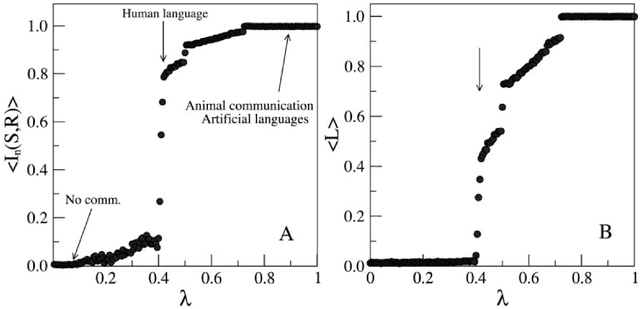


Zipf's law predicts a power-law relationship between word rank and frequency in language communication systems and has been widely reported in a variety of natural language processing applications. However, the emergence of natural language is often modeled as a function of bias between speaker and listener interests, which lacks a direct way of relating information-theoretic bias to Zipfian rank. A function of bias also serves as an unintuitive interpretation of the communicative effort exchanged between a speaker and a listener. We counter these shortcomings by proposing a novel integral transform and kernel for mapping communicative bias functions to corresponding word frequency-rank representations at any arbitrary phase transition point, resulting in a direct way to link communicative effort (modeled by speaker/listener bias) to specific vocabulary used (represented by word rank). We demonstrate the practical utility of our integral transform by showing how a change from bias to rank results in greater accuracy and performance at an image classification task for assigning word labels to images randomly subsampled from CIFAR10. We model this task as a reinforcement learning game between a speaker and listener and compare the relative impact of bias and Zipfian word rank on communicative performance (and accuracy) between the two agents.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge