Mixture Model Averaging for Clustering
Paper and Code
Jul 26, 2014
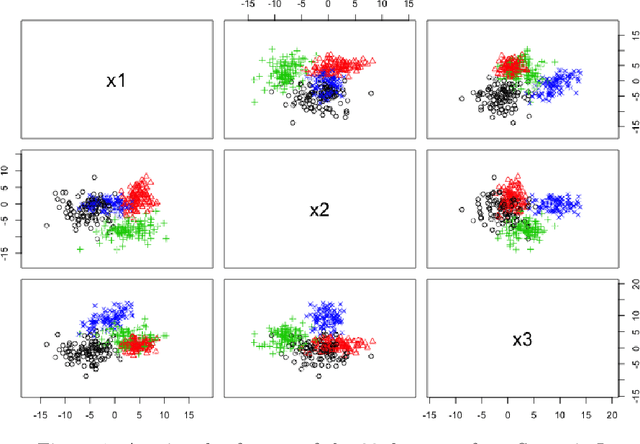

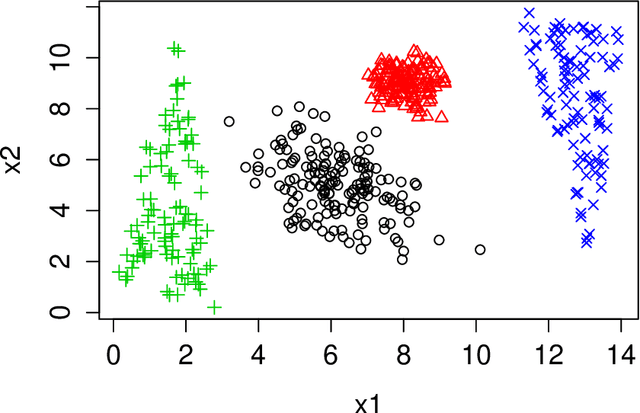
In mixture model-based clustering applications, it is common to fit several models from a family and report clustering results from only the `best' one. In such circumstances, selection of this best model is achieved using a model selection criterion, most often the Bayesian information criterion. Rather than throw away all but the best model, we average multiple models that are in some sense close to the best one, thereby producing a weighted average of clustering results. Two (weighted) averaging approaches are considered: averaging the component membership probabilities and averaging models. In both cases, Occam's window is used to determine closeness to the best model and weights are computed within a Bayesian model averaging paradigm. In some cases, we need to merge components before averaging; we introduce a method for merging mixture components based on the adjusted Rand index. The effectiveness of our model-based clustering averaging approaches is illustrated using a family of Gaussian mixture models on real and simulated data.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge