Mitigating the Curse of Correlation in Security Games by Entropy Maximization
Paper and Code
Jan 03, 2018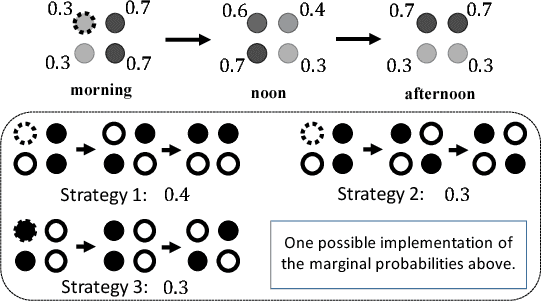
In Stackelberg security games, a defender seeks to randomly allocate limited security resources to protect critical targets from an attack. In this paper, we study a fundamental, yet underexplored, phenomenon in security games, which we term the \emph{Curse of Correlation} (CoC). Specifically, we observe that there are inevitable correlations among the protection status of different targets. Such correlation is a crucial concern, especially in \emph{spatio-temporal} domains like conservation area patrolling, where attackers can surveil patrollers at certain areas and then infer their patrolling routes using such correlations. To mitigate this issue, we propose to design entropy-maximizing defending strategies for spatio-temporal security games, which frequently suffer from CoC. We prove that the problem is \#P-hard in general. However, it admits efficient algorithms in well-motivated special settings. Our experiments show significant advantages of max-entropy algorithms over previous algorithms. A scalable implementation of our algorithm is currently under pre-deployment testing for integration into FAMS software to improve the scheduling of US federal air marshals.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge