Learning Multi-Layered GBDT Via Back Propagation
Paper and Code
Sep 27, 2021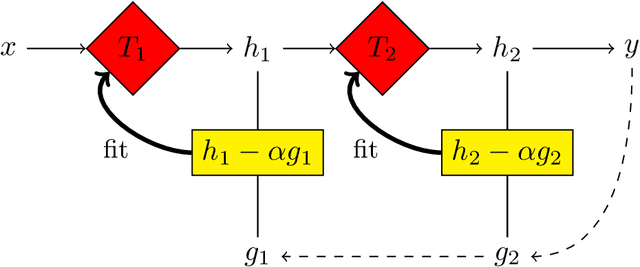
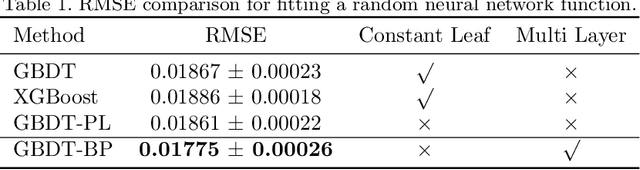
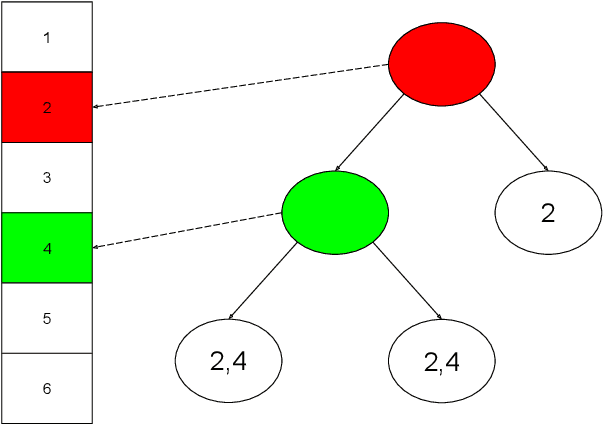
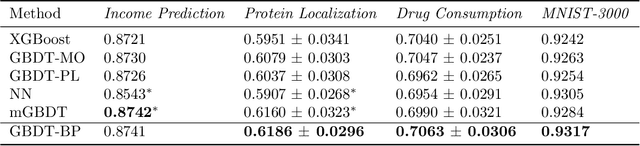
Deep neural networks are able to learn multi-layered representation via back propagation (BP). Although the gradient boosting decision tree (GBDT) is effective for modeling tabular data, it is non-differentiable with respect to its input, thus suffering from learning multi-layered representation. In this paper, we propose a framework of learning multi-layered GBDT via BP. We approximate the gradient of GBDT based on linear regression. Specifically, we use linear regression to replace the constant value at each leaf ignoring the contribution of individual samples to the tree structure. In this way, we estimate the gradient for intermediate representations, which facilitates BP for multi-layered GBDT. Experiments show the effectiveness of the proposed method in terms of performance and representation ability. To the best of our knowledge, this is the first work of optimizing multi-layered GBDT via BP. This work provides a new possibility of exploring deep tree based learning and combining GBDT with neural networks.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge