Learning Direct and Inverse Transmission Matrices
Paper and Code
Jan 15, 2019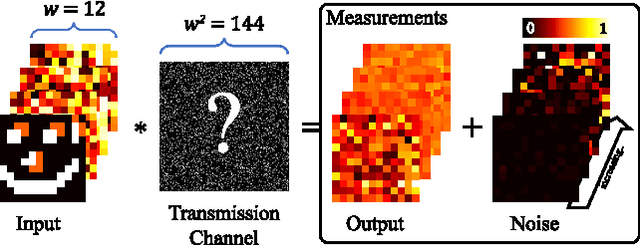
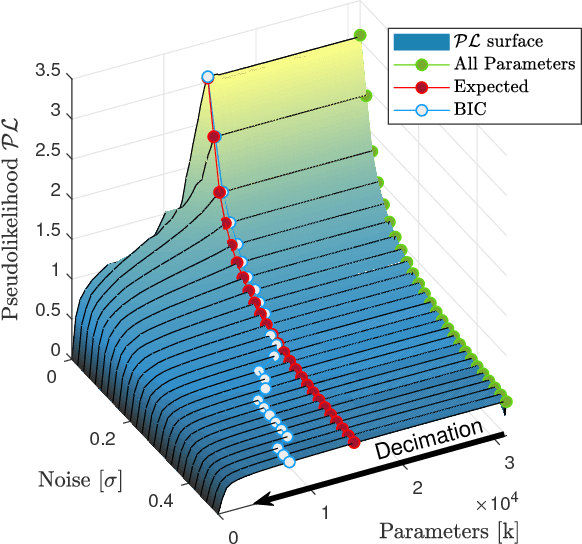
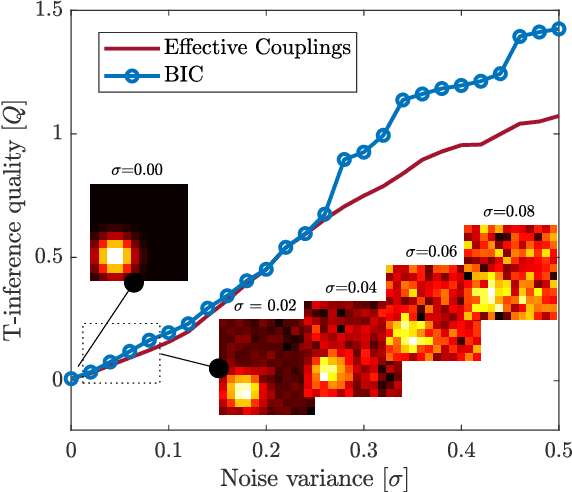
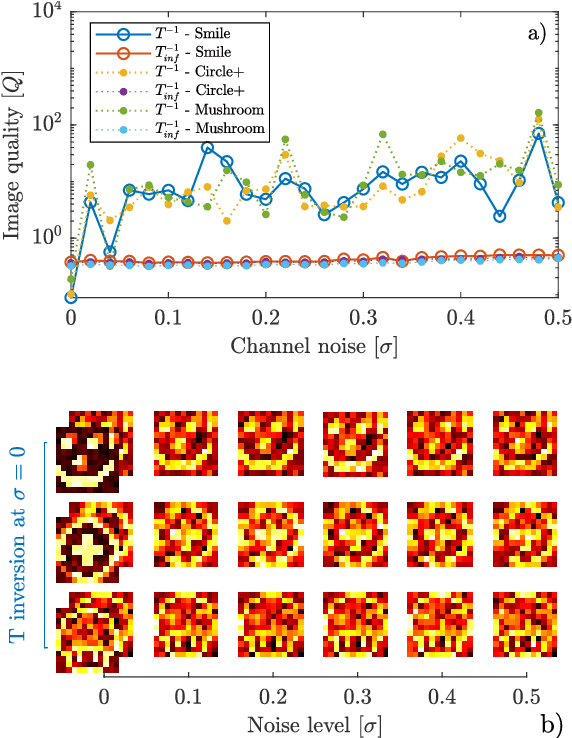
Linear problems appear in a variety of disciplines and their application for the transmission matrix recovery is one of the most stimulating challenges in biomedical imaging. Its knowledge turns any random media into an optical tool that can focus or transmit an image through disorder. Here, converting an input-output problem into a statistical mechanical formulation, we investigate how inference protocols can learn the transmission couplings by pseudolikelihood maximization. Bridging linear regression and thermodynamics let us propose an innovative framework to pursue the solution of the scattering-riddle.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge