Knowledge Patterns
Paper and Code
May 08, 2020
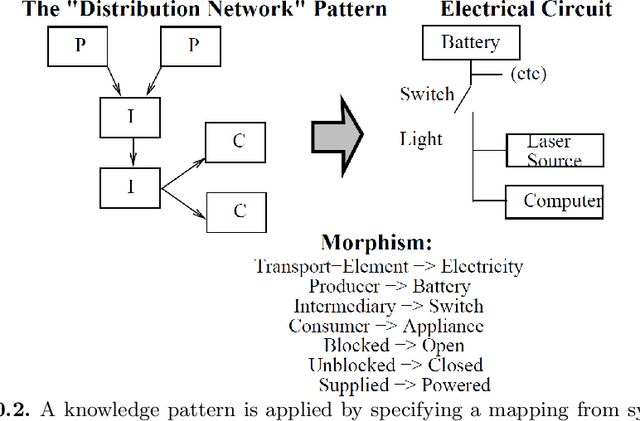
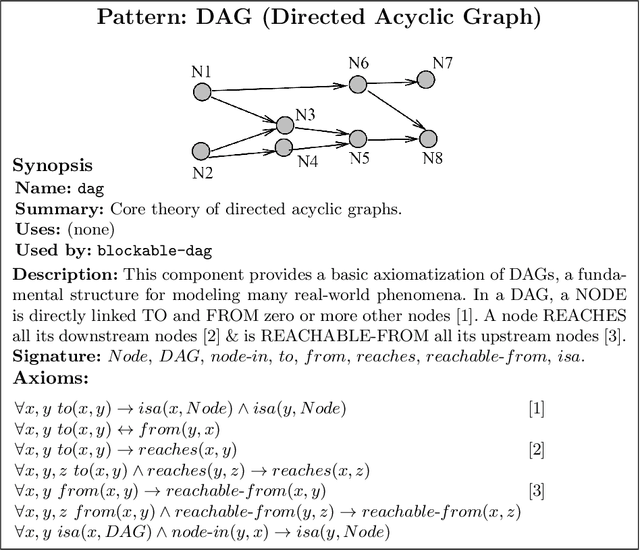

This paper describes a new technique, called "knowledge patterns", for helping construct axiom-rich, formal ontologies, based on identifying and explicitly representing recurring patterns of knowledge (theory schemata) in the ontology, and then stating how those patterns map onto domain-specific concepts in the ontology. From a modeling perspective, knowledge patterns provide an important insight into the structure of a formal ontology: rather than viewing a formal ontology simply as a list of terms and axioms, knowledge patterns views it as a collection of abstract, modular theories (the "knowledge patterns") plus a collection of modeling decisions stating how different aspects of the world can be modeled using those theories. Knowledge patterns make both those abstract theories and their mappings to the domain of interest explicit, thus making modeling decisions clear, and avoiding some of the ontological confusion that can otherwise arise. In addition, from a computational perspective, knowledge patterns provide a simple and computationally efficient mechanism for facilitating knowledge reuse. We describe the technique and an application built using them, and then critique its strengths and weaknesses. We conclude that this technique enables us to better explicate both the structure and modeling decisions made when constructing a formal axiom-rich ontology.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge