Imaging with Rays: Microscopy, Medical Imaging, and Computer Vision
Paper and Code
Feb 11, 2014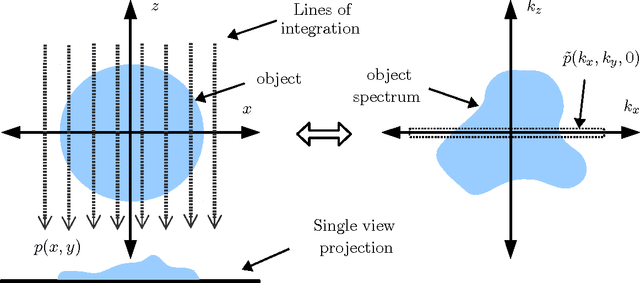
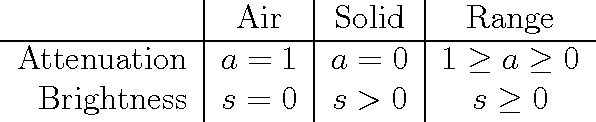
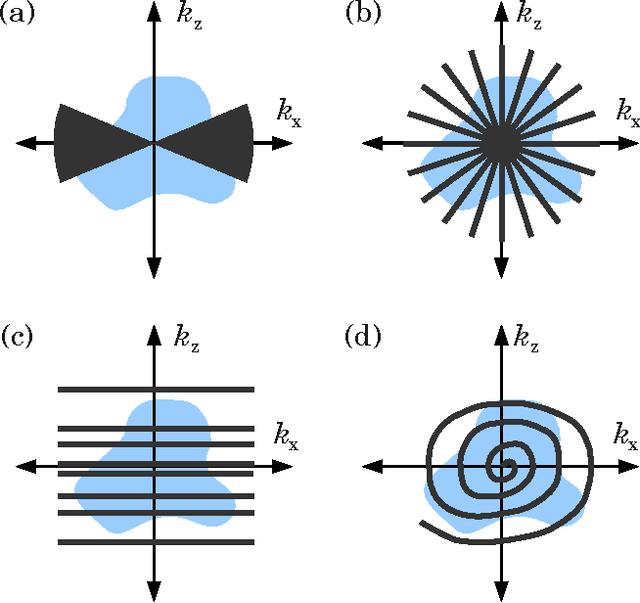
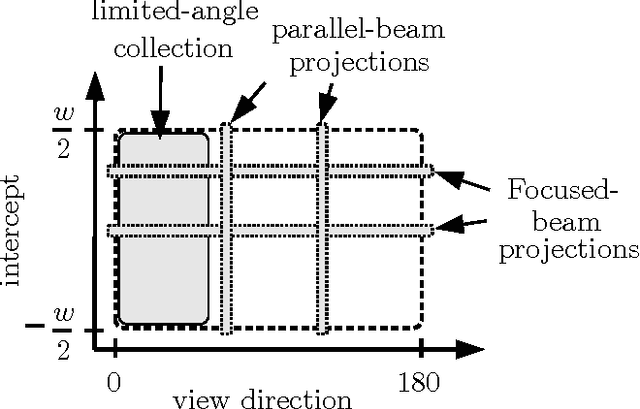
In this paper we broadly consider techniques which utilize projections on rays for data collection, with particular emphasis on optical techniques. We formulate a variety of imaging techniques as either special cases or extensions of tomographic reconstruction. We then consider how the techniques must be extended to describe objects containing occlusion, as with a self-occluding opaque object. We formulate the reconstruction problem as a regularized nonlinear optimization problem to simultaneously solve for object brightness and attenuation, where the attenuation can become infinite. We demonstrate various simulated examples for imaging opaque objects, including sparse point sources, a conventional multiview reconstruction technique, and a super-resolving technique which exploits occlusion to resolve an image.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge