How Does Frequency Bias Affect the Robustness of Neural Image Classifiers against Common Corruption and Adversarial Perturbations?
Paper and Code
May 09, 2022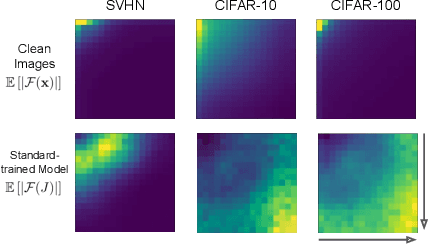
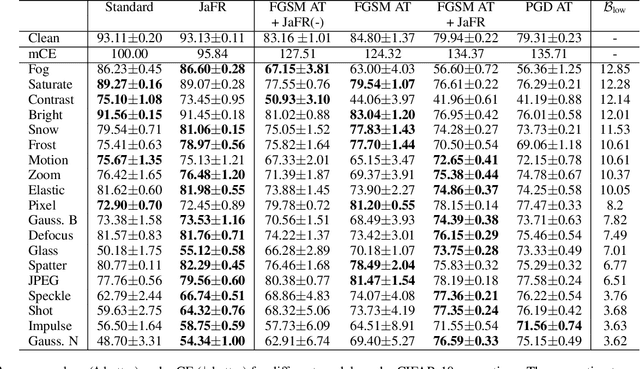
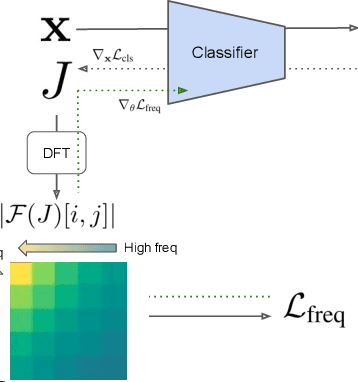
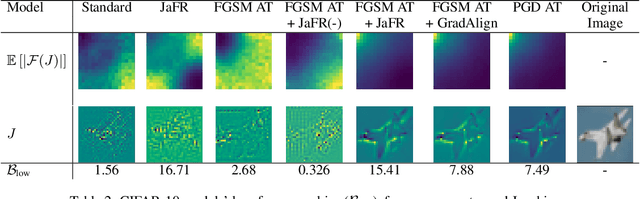
Model robustness is vital for the reliable deployment of machine learning models in real-world applications. Recent studies have shown that data augmentation can result in model over-relying on features in the low-frequency domain, sacrificing performance against low-frequency corruptions, highlighting a connection between frequency and robustness. Here, we take one step further to more directly study the frequency bias of a model through the lens of its Jacobians and its implication to model robustness. To achieve this, we propose Jacobian frequency regularization for models' Jacobians to have a larger ratio of low-frequency components. Through experiments on four image datasets, we show that biasing classifiers towards low (high)-frequency components can bring performance gain against high (low)-frequency corruption and adversarial perturbation, albeit with a tradeoff in performance for low (high)-frequency corruption. Our approach elucidates a more direct connection between the frequency bias and robustness of deep learning models.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge