Geometry of Graph Edit Distance Spaces
Paper and Code
May 29, 2015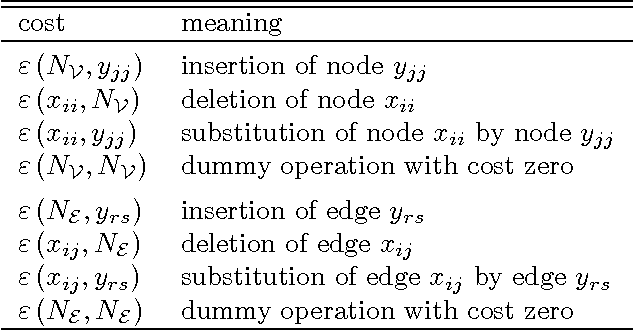
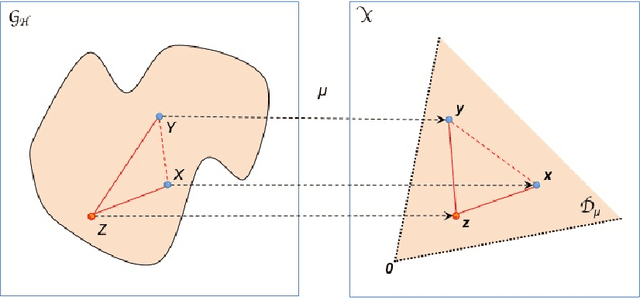
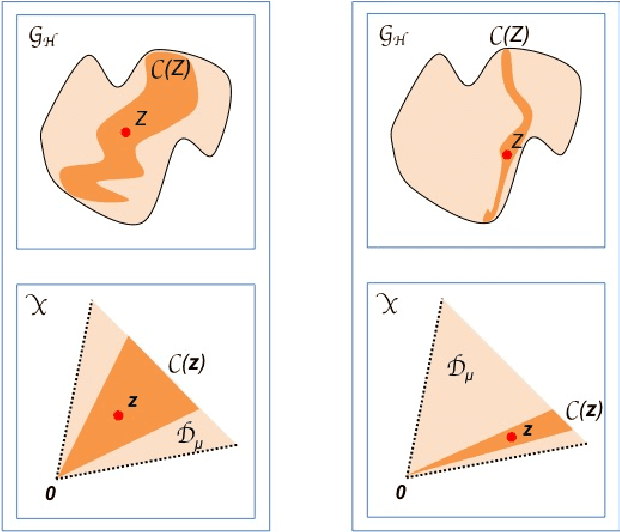
In this paper we study the geometry of graph spaces endowed with a special class of graph edit distances. The focus is on geometrical results useful for statistical pattern recognition. The main result is the Graph Representation Theorem. It states that a graph is a point in some geometrical space, called orbit space. Orbit spaces are well investigated and easier to explore than the original graph space. We derive a number of geometrical results from the orbit space representation, translate them to the graph space, and indicate their significance and usefulness in statistical pattern recognition.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge