Fractional Order Load-Frequency Control of Interconnected Power Systems Using Chaotic Multi-objective Optimization

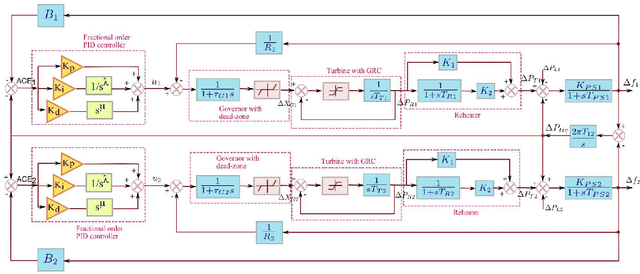
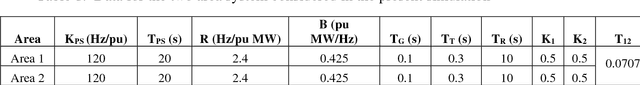
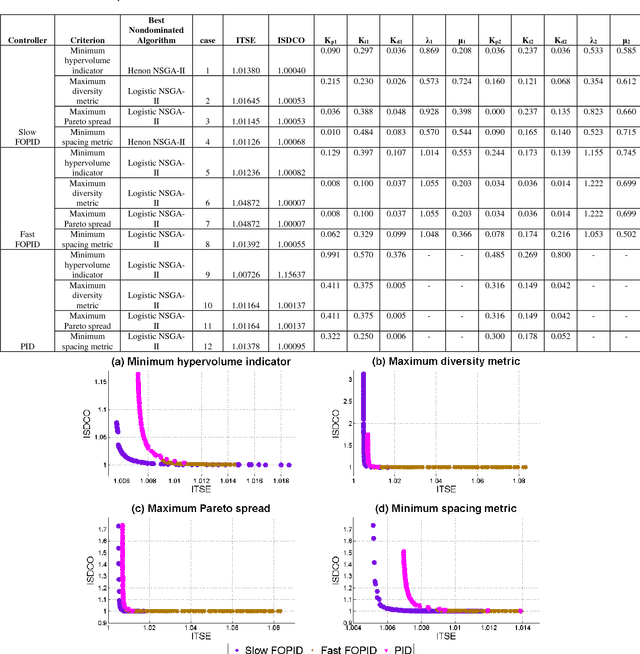
Fractional order proportional-integral-derivative (FOPID) controllers are designed for load frequency control (LFC) of two interconnected power systems. Conflicting time domain design objectives are considered in a multi objective optimization (MOO) based design framework to design the gains and the fractional differ-integral orders of the FOPID controllers in the two areas. Here, we explore the effect of augmenting two different chaotic maps along with the uniform random number generator (RNG) in the popular MOO algorithm - the Non-dominated Sorting Genetic Algorithm-II (NSGA-II). Different measures of quality for MOO e.g. hypervolume indicator, moment of inertia based diversity metric, total Pareto spread, spacing metric are adopted to select the best set of controller parameters from multiple runs of all the NSGA-II variants (i.e. nominal and chaotic versions). The chaotic versions of the NSGA-II algorithm are compared with the standard NSGA-II in terms of solution quality and computational time. In addition, the Pareto optimal fronts showing the trade-off between the two conflicting time domain design objectives are compared to show the advantage of using the FOPID controller over that with simple PID controller. The nature of fast/slow and high/low noise amplification effects of the FOPID structure or the four quadrant operation in the two inter-connected areas of the power system is also explored. A fuzzy logic based method has been adopted next to select the best compromise solution from the best Pareto fronts corresponding to each MOO comparison criteria. The time domain system responses are shown for the fuzzy best compromise solutions under nominal operating conditions. Comparative analysis on the merits and de-merits of each controller structure is reported then. A robustness analysis is also done for the PID and the FOPID controllers.