Exponential Negation of a Probability Distribution

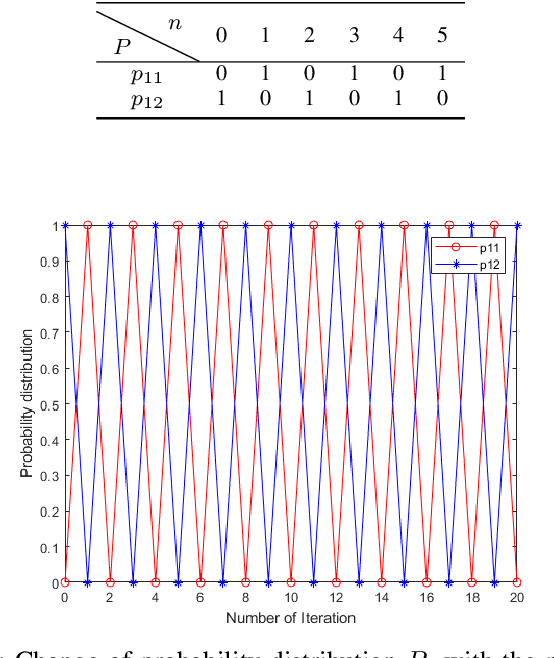
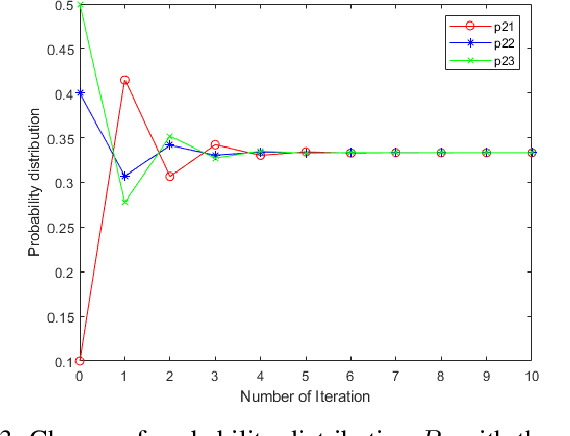
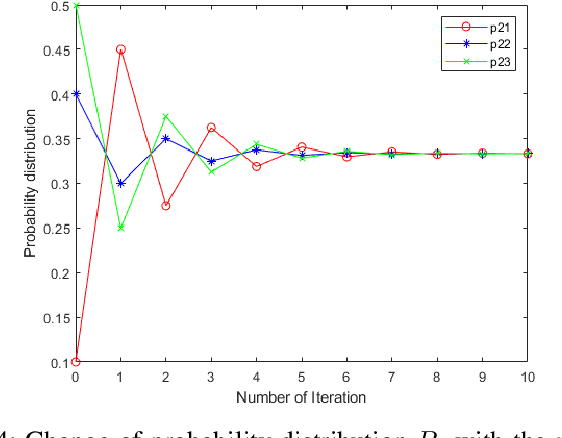
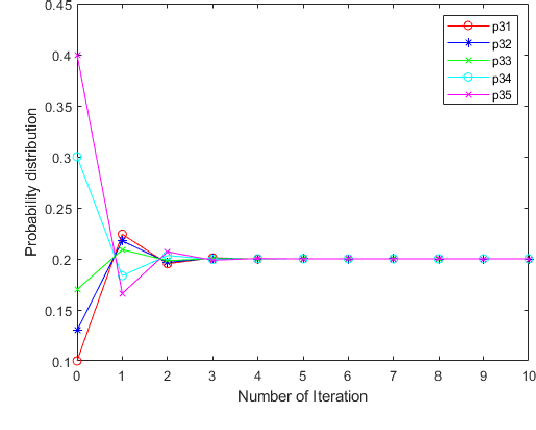
Negation operation is important in intelligent information processing. Different with existing arithmetic negation, an exponential negation is presented in this paper. The new negation can be seen as a kind of geometry negation. Some basic properties of the proposed negation is investigated, we find that the fix point is the uniform probability distribution.The negation is an entropy increase operation and all the probability distributions will converge to the uniform distribution after multiple negation iterations. The number of iterations of convergence is inversely proportional to the number of elements in the distribution. Some numerical examples are used to illustrate the efficiency of the proposed negation.