Environmental Information Improves Robotic Search Performance
Paper and Code
Jun 22, 2018


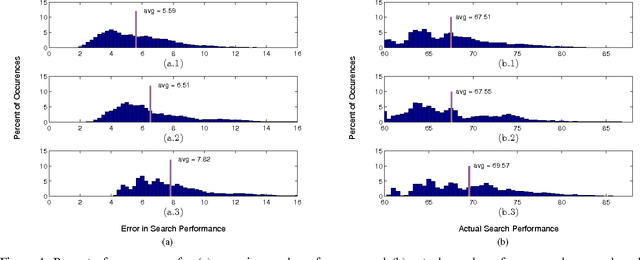
We address the problem where a mobile search agent seeks to find an unknown number of stationary objects distributed in a bounded search domain, and the search mission is subject to time/distance constraint. Our work accounts for false positives, false negatives and environmental uncertainty. We consider the case that the performance of a search sensor is dependent on the environment (e.g., clutter density), and therefore sensor performance is better in some locations than in others. We specifically consider applications where environmental information can be acquired either by a separate vehicle or by the same vehicle that performs the search task. Our main contribution in this study is to formally derive a decision-theoretic cost function to compute the locations where the environmental information should be acquired. For the cases where computing the optimal locations to sample the environment is computationally expensive, we offer an approximation approach that yields provable near-optimal paths. We show that our decision-theoretic cost function outperforms the information-maximization approach, which is often employed in similar applications.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge