Embedding Knowledge Graphs Based on Transitivity and Antisymmetry of Rules
Paper and Code
Apr 19, 2017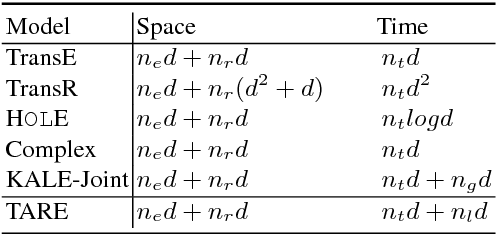
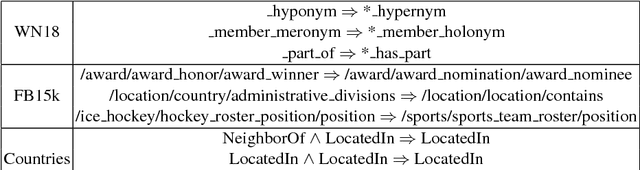


Representation learning of knowledge graphs encodes entities and relation types into a continuous low-dimensional vector space, learns embeddings of entities and relation types. Most existing methods only concentrate on knowledge triples, ignoring logic rules which contain rich background knowledge. Although there has been some work aiming at leveraging both knowledge triples and logic rules, they ignore the transitivity and antisymmetry of logic rules. In this paper, we propose a novel approach to learn knowledge representations with entities and ordered relations in knowledges and logic rules. The key idea is to integrate knowledge triples and logic rules, and approximately order the relation types in logic rules to utilize the transitivity and antisymmetry of logic rules. All entries of the embeddings of relation types are constrained to be non-negative. We translate the general constrained optimization problem into an unconstrained optimization problem to solve the non-negative matrix factorization. Experimental results show that our model significantly outperforms other baselines on knowledge graph completion task. It indicates that our model is capable of capturing the transitivity and antisymmetry information, which is significant when learning embeddings of knowledge graphs.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge