Edge-aware Bidirectional Diffusion for Dense Depth Estimation from Light Fields
Paper and Code
Jul 07, 2021
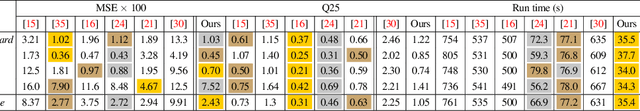
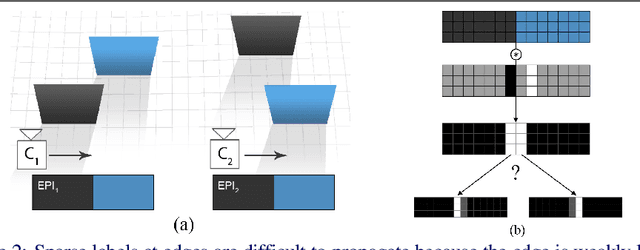
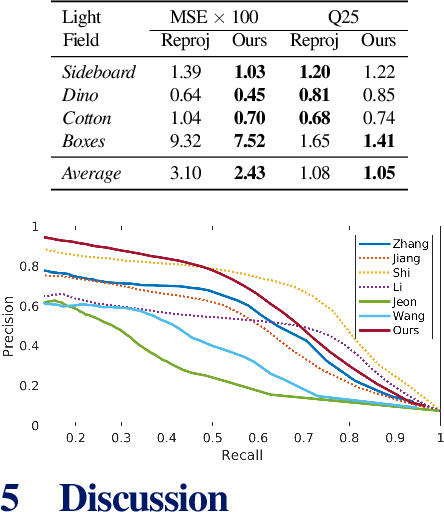
We present an algorithm to estimate fast and accurate depth maps from light fields via a sparse set of depth edges and gradients. Our proposed approach is based around the idea that true depth edges are more sensitive than texture edges to local constraints, and so they can be reliably disambiguated through a bidirectional diffusion process. First, we use epipolar-plane images to estimate sub-pixel disparity at a sparse set of pixels. To find sparse points efficiently, we propose an entropy-based refinement approach to a line estimate from a limited set of oriented filter banks. Next, to estimate the diffusion direction away from sparse points, we optimize constraints at these points via our bidirectional diffusion method. This resolves the ambiguity of which surface the edge belongs to and reliably separates depth from texture edges, allowing us to diffuse the sparse set in a depth-edge and occlusion-aware manner to obtain accurate dense depth maps.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge