Dimensionless Policies based on the Buckingham $π$ Theorem: Is it a good way to Generalize Numerical Results?
Paper and Code
Jul 29, 2023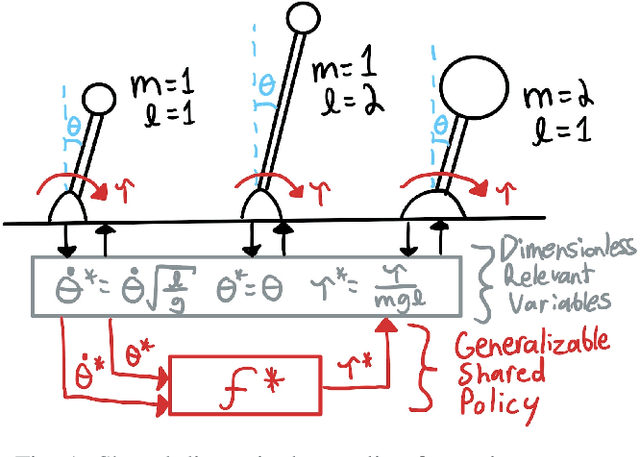
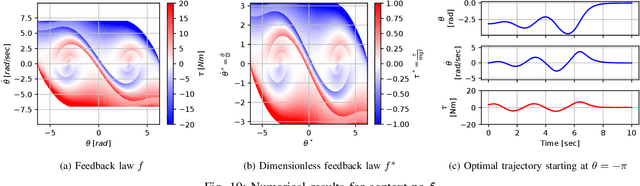
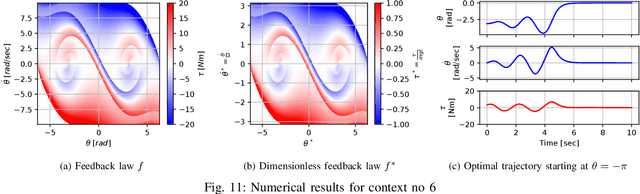
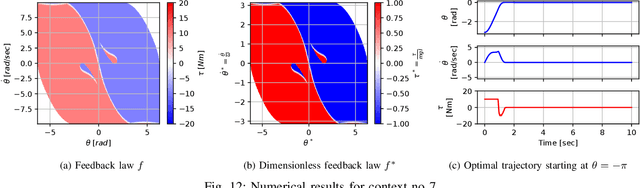
Yes if the context, the list of variables defining the motion control problem, is dimensionally similar. Here we show that by modifying the problem formulation using dimensionless variables, we can re-use the optimal control law generated numerically for a specific system to a sub-space of dimensionally similar systems. This is demonstrated, with numerically generated optimal controllers, for the classic motion control problem of swinging-up a torque-limited inverted pendulum. We also discuss the concept of regime, a region in the space of context variables, that can help relax the condition on dimensional similarity. Futhermore, we discuss how applying dimensionnal scaling of the input and output of a context-specific policy is equivalent to substituing the new systems parameters in an analytical equation for dimentionnaly similar systems. It remains to be seen if this approach can also help generalizing policies for more complex high-dimensional problems.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge