Degrees of Freedom and Model Selection for kmeans Clustering

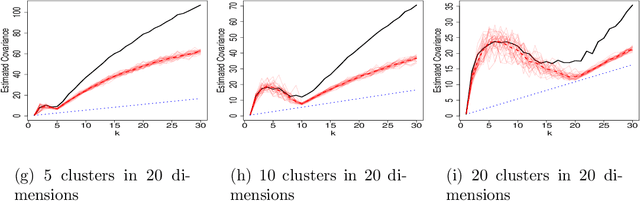
This paper investigates the problem of model selection for kmeans clustering, based on conservative estimates of the model degrees of freedom. An extension of Stein's lemma, which is used in unbiased risk estimation, is used to obtain an expression which allows one to approximate the degrees of freedom. Empirically based estimates of this approximation are obtained. The degrees of freedom estimates are then used within the popular Bayesian Information Criterion to perform model selection. The proposed estimation procedure is validated in a thorough simulation study, and the robustness is assessed through relaxations of the modelling assumptions and on data from real applications. Comparisons with popular existing techniques suggest that this approach performs extremely well when the modelling assumptions