DCT-based Air Interface Design for Function Computation
Paper and Code
Jun 28, 2023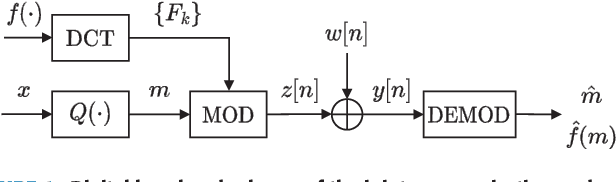
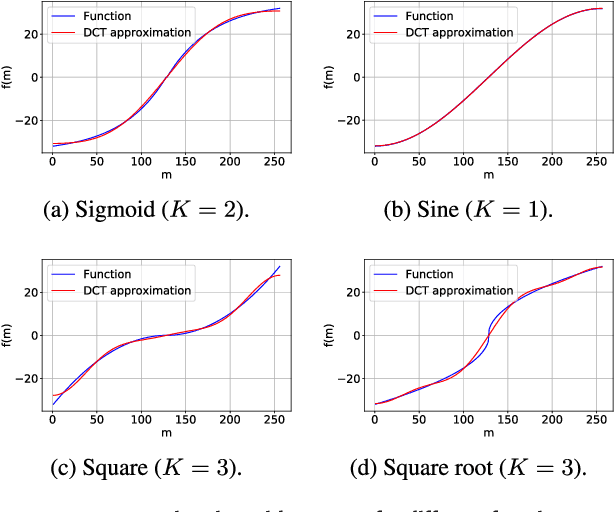
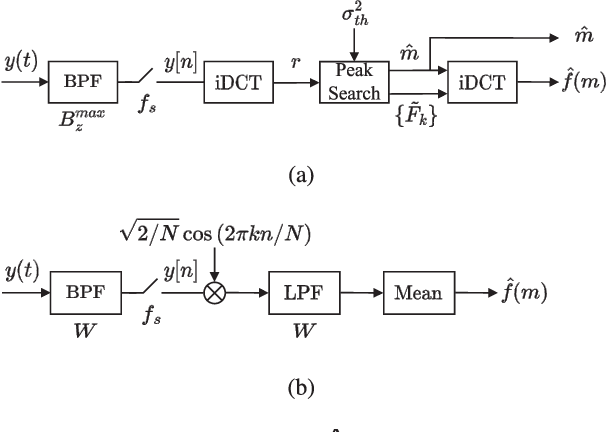
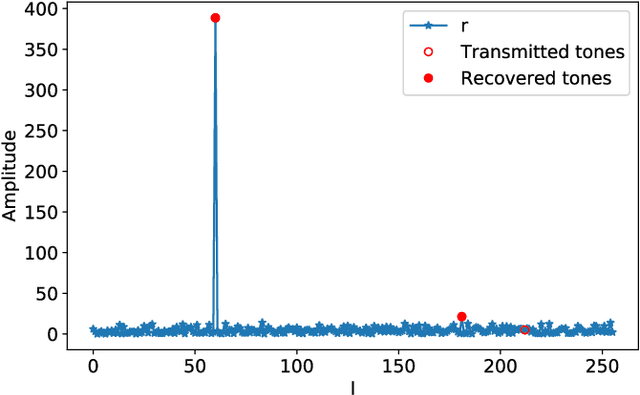
With the integration of communication and computing, it is expected that part of the computing is transferred to the transmitter side. In this paper we address the general problem of Frequency Modulation (FM) for function approximation through a communication channel. We exploit the benefits of the Discrete Cosine Transform (DCT) to approximate the function and design the waveform. In front of other approximation schemes, the DCT uses basis of controlled dynamic, which is a desirable property for a practical implementation. Furthermore, the proposed modulation allows to recover both the measurement and the function in a single transmission. Our experiments show that this scheme outperforms the double side-band (DSB) modulation in terms of mean squared error (MSE). This can also be implemented with an agnostic receiver, in which the function is unknown to the receiver. Finally, the proposed modulation is compatible with some of the existing transmission technologies for sensor networks.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge