Data-Driven Parameter Estimation
Paper and Code
Jan 29, 2022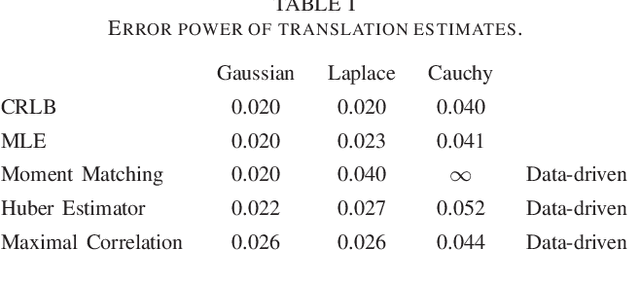
Optimum parameter estimation methods require knowledge of a parametric probability density that statistically describes the available observations. In this work we examine Bayesian and non-Bayesian parameter estimation problems under a data-driven formulation where the necessary parametric probability density is replaced by available data. We present various data-driven versions that either result in neural network approximations of the optimum estimators or in well defined optimization problems that can be solved numerically. In particular, for the data-driven equivalent of non-Bayesian estimation we end up with optimization problems similar to the ones encountered for the design of generative networks.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge