Binary Spatial Random Field Reconstruction from Non-Gaussian Inhomogeneous Time-series Observations
Paper and Code
Apr 07, 2022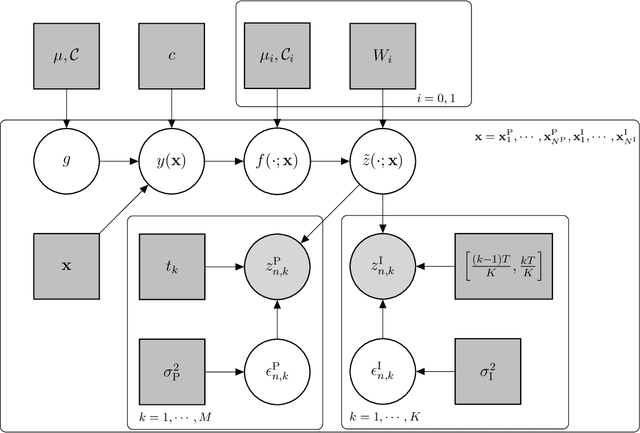
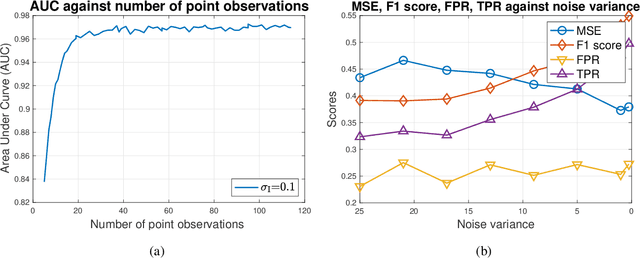
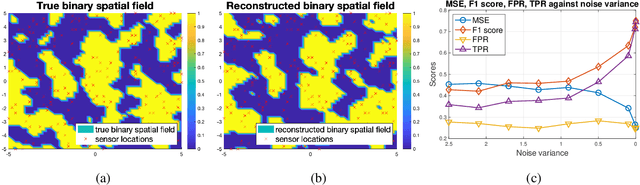
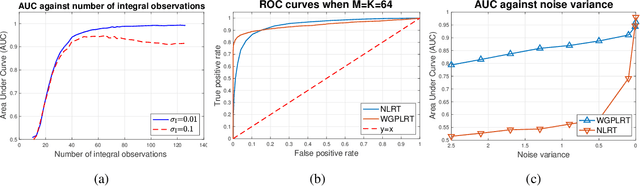
We develop a new model for binary spatial random field reconstruction of a physical phenomenon which is partially observed via inhomogeneous time-series data. We consider a sensor network deployed over a vast geographical region where sensors observe temporal processes and transmit compressed observations to the Fusion Center (FC). Two types of sensors are considered; one collects point observations at specific time points while the other collects integral observations over time intervals. Subsequently, the FC uses the compressed observations to infer the spatial phenomenon modeled as a binary spatial random field. We show that the resulting posterior predictive distribution is intractable and develop a tractable two-step procedure to perform inference. First, we develop procedures to approximately perform Likelihood Ratio Tests on the time-series data, for both point sensors and integral sensors, in order to compress the temporal observations to a single bit. Second, after the compressed observations are transmitted to the FC, we develop a Spatial Best Linear Unbiased Estimator (S-BLUE) in order for the FC to reconstruct the binary spatial random field at an arbitrary spatial location. Finally, we present a comprehensive study of the performance of the proposed approaches using both synthetic and real-world experiments. A weather dataset from the National Environment Agency (NEA) of Singapore with fields including temperature and relative humidity is used in the real-world experiments to validate the proposed approaches.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge