Approximate exploitability: Learning a best response in large games

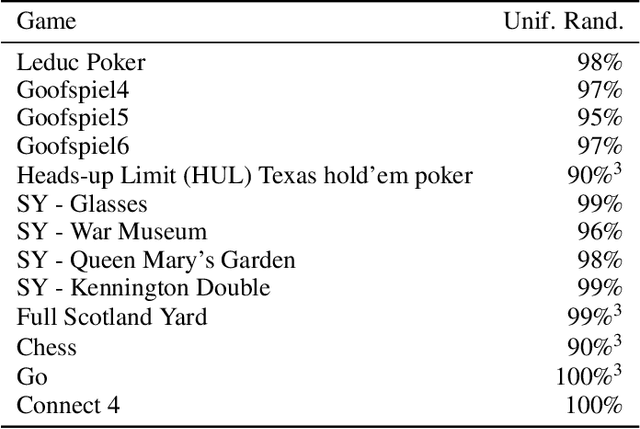
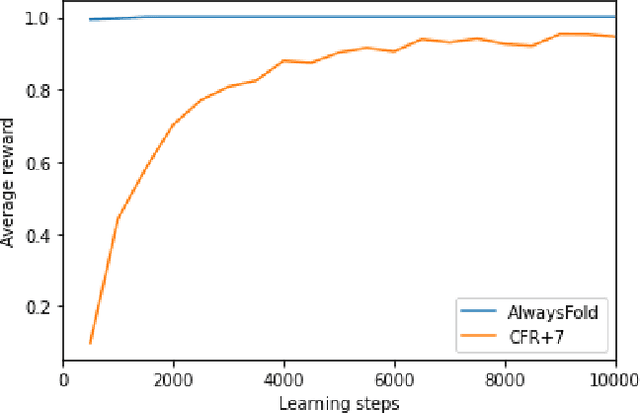
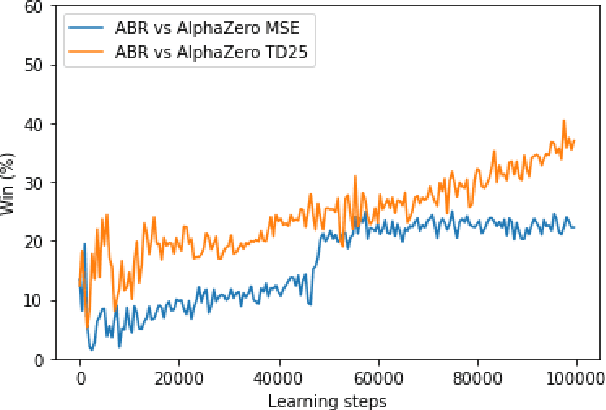
A common metric in games of imperfect information is exploitability, i.e. the performance of a policy against the worst-case opponent. This metric has many nice properties, but is intractable to compute in large games as it requires a full search of the game tree to calculate a best response to the given policy. We introduce a new metric, approximate exploitability, that calculates an analogous metric to exploitability using an approximate best response. This method scales to large games with tractable belief spaces. We focus only on the two-player, zero-sum case. Additionally, we provide empirical results for a specific instance of the method, demonstrating that it can effectively exploit agents in large games. We demonstrate that our method converges to exploitability in the tabular setting and the function approximation setting for small games, and demonstrate that it can consistently find exploits for weak policies in large games, showing results on Chess, Go, Heads-up No Limit Texas Hold'em, and other games.