Amplitude-to-Phase Conversion in Injection-Locked CMOS Ring Oscillators
Paper and Code
Dec 28, 2024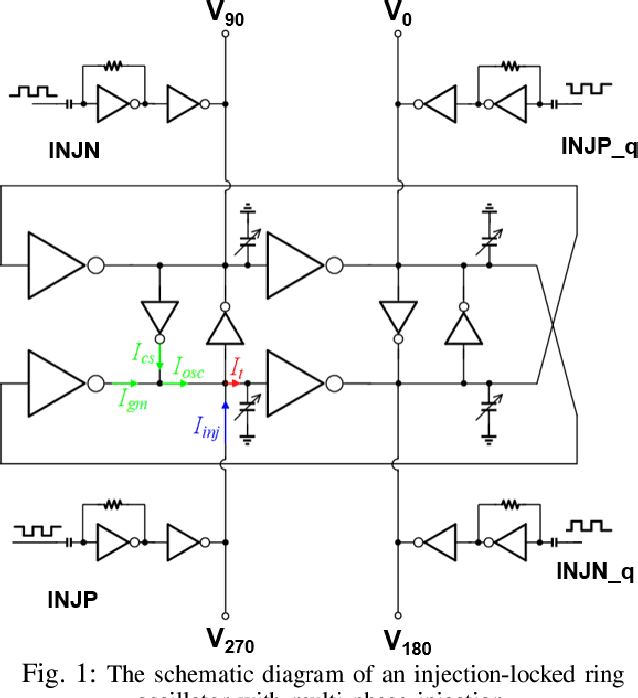
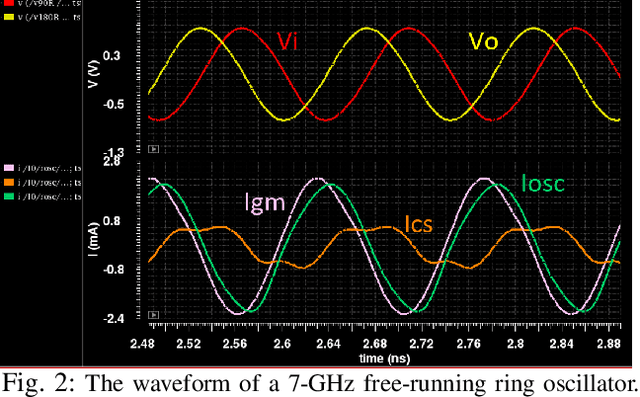
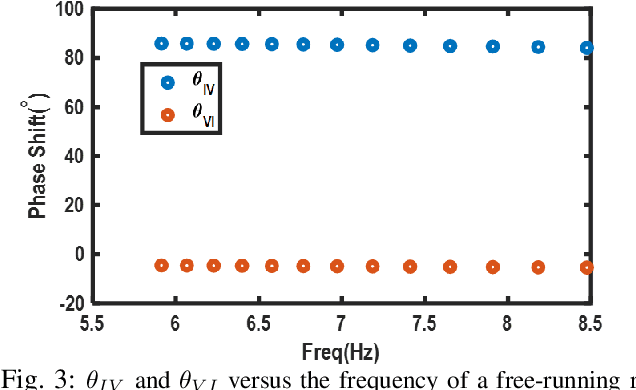
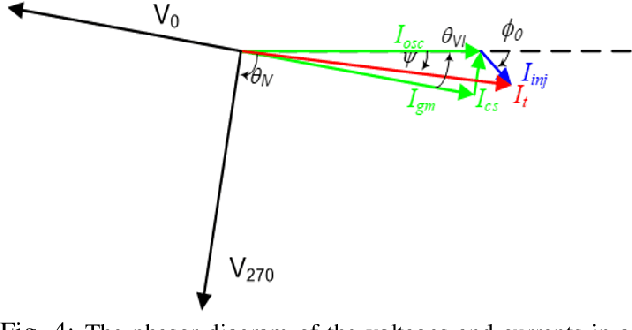
Injection-locked ring oscillators (ILROs) are extensively employed for multi-phase clock generation in wireline and optical links. However, existing injection-locking theorems primarily rely on linearized phase-domain or nonlinear time-domain models, which fail to account for amplitude-to-phase conversion effects inherent in ILROs. This paper introduces an enhanced analytical model based on an extension of Adler's equation, explicitly incorporating amplitude-to-phase conversion. Simulation results demonstrate strong alignment with the proposed analytical predictions, validating the model's accuracy in capturing the locking range and phasor relationships.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge