Almost Optimal Proper Learning and Testing Polynomials
Paper and Code
Feb 07, 2022

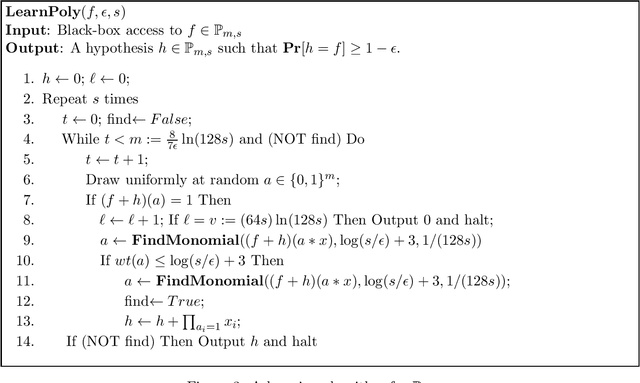
We give the first almost optimal polynomial-time proper learning algorithm of Boolean sparse multivariate polynomial under the uniform distribution. For $s$-sparse polynomial over $n$ variables and $\epsilon=1/s^\beta$, $\beta>1$, our algorithm makes $$q_U=\left(\frac{s}{\epsilon}\right)^{\frac{\log \beta}{\beta}+O(\frac{1}{\beta})}+ \tilde O\left(s\right)\left(\log\frac{1}{\epsilon}\right)\log n$$ queries. Notice that our query complexity is sublinear in $1/\epsilon$ and almost linear in $s$. All previous algorithms have query complexity at least quadratic in $s$ and linear in $1/\epsilon$. We then prove the almost tight lower bound $$q_L=\left(\frac{s}{\epsilon}\right)^{\frac{\log \beta}{\beta}+\Omega(\frac{1}{\beta})}+ \Omega\left(s\right)\left(\log\frac{1}{\epsilon}\right)\log n,$$ Applying the reduction in~\cite{Bshouty19b} with the above algorithm, we give the first almost optimal polynomial-time tester for $s$-sparse polynomial. Our tester, for $\beta>3.404$, makes $$\tilde O\left(\frac{s}{\epsilon}\right)$$ queries.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge