Accelerated Alternating Minimization for X-ray Tomographic Reconstruction
Paper and Code
Aug 02, 2021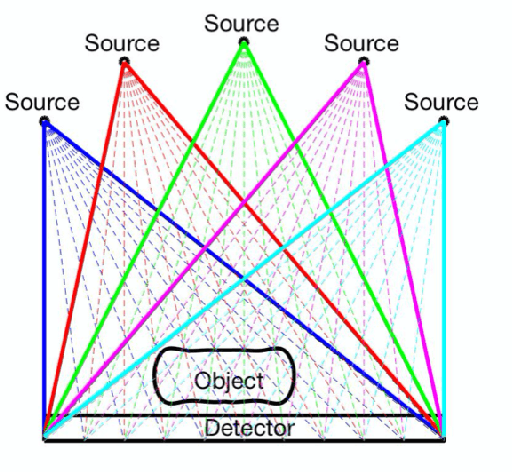


While Computerized Tomography (CT) images can help detect disease such as Covid-19, regular CT machines are large and expensive. Cheaper and more portable machines suffer from errors in geometry acquisition that downgrades CT image quality. The errors in geometry can be represented with parameters in the mathematical model for image reconstruction. To obtain a good image, we formulate a nonlinear least squares problem that simultaneously reconstructs the image and corrects for errors in the geometry parameters. We develop an accelerated alternating minimization scheme to reconstruct the image and geometry parameters.
* 18 pages, 14 figures, submitted to SIURO
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge