A Numerical Example on the Principles of Stochastic Discrimination

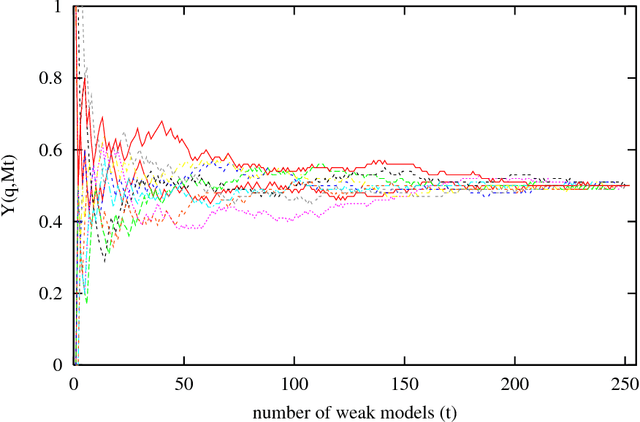
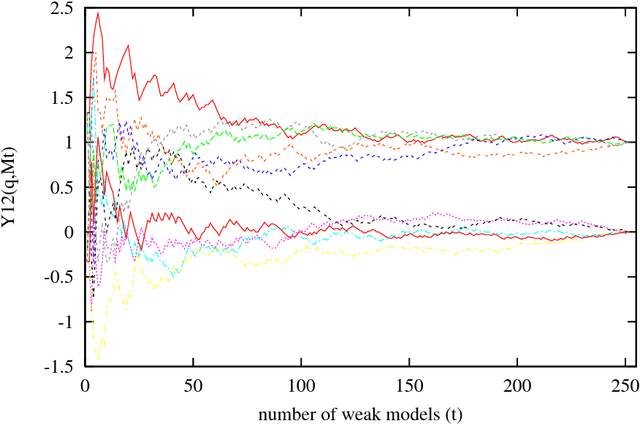
Studies on ensemble methods for classification suffer from the difficulty of modeling the complementary strengths of the components. Kleinberg's theory of stochastic discrimination (SD) addresses this rigorously via mathematical notions of enrichment, uniformity, and projectability of an ensemble. We explain these concepts via a very simple numerical example that captures the basic principles of the SD theory and method. We focus on a fundamental symmetry in point set covering that is the key observation leading to the foundation of the theory. We believe a better understanding of the SD method will lead to developments of better tools for analyzing other ensemble methods.