A modified Physarum-inspired model for the user equilibrium traffic assignment problem
Paper and Code
Dec 19, 2016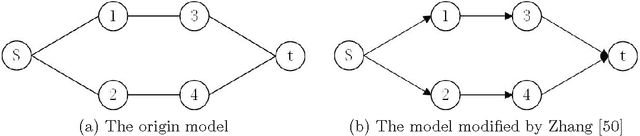
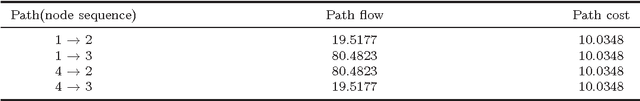
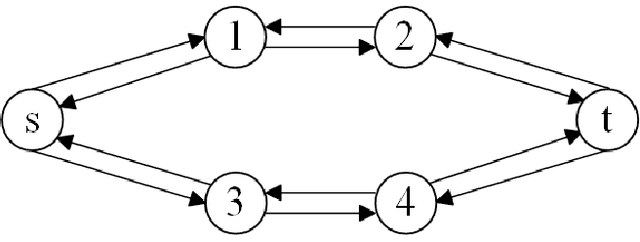
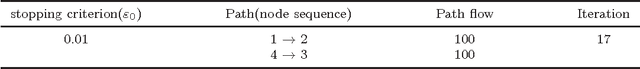
The user equilibrium traffic assignment principle is very important in the traffic assignment problem. Mathematical programming models are designed to solve the user equilibrium problem in traditional algorithms. Recently, the Physarum shows the ability to address the user equilibrium and system optimization traffic assignment problems. However, the Physarum model are not efficient in real traffic networks with two-way traffic characteristics and multiple origin-destination pairs. In this article, a modified Physarum-inspired model for the user equilibrium problem is proposed. By decomposing traffic flux based on origin nodes, the traffic flux from different origin-destination pairs can be distinguished in the proposed model. The Physarum can obtain the equilibrium traffic flux when no shorter path can be discovered between each origin-destination pair. Finally, numerical examples use the Sioux Falls network to demonstrate the rationality and convergence properties of the proposed model.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge