Deep Neural Network-Based Prediction of B-Cell Epitopes for SARS-CoV and SARS-CoV-2: Enhancing Vaccine Design through Machine Learning

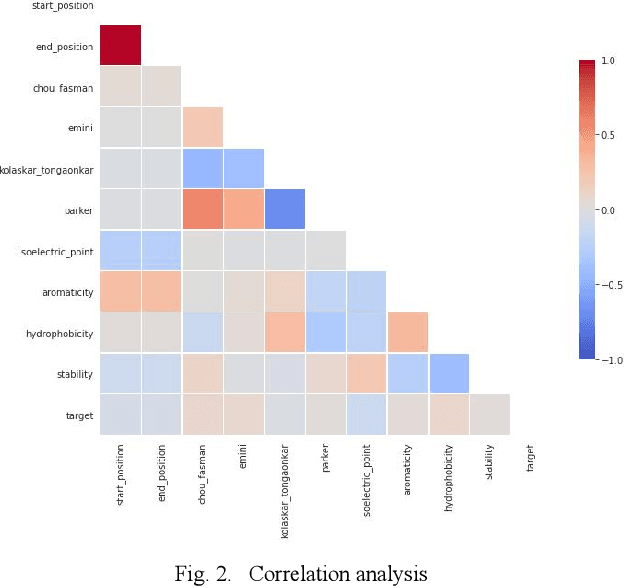
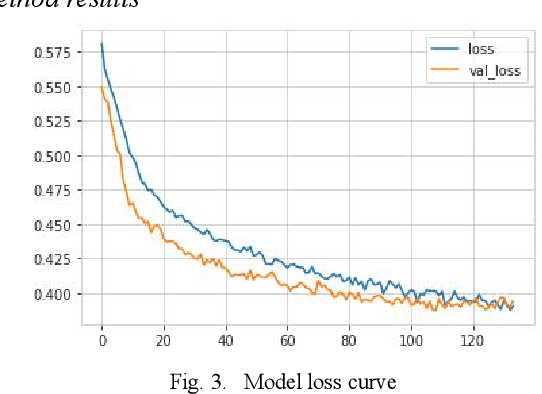
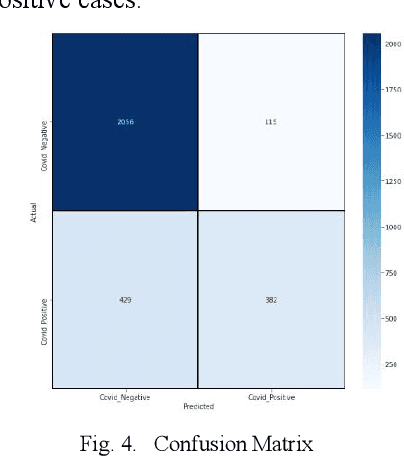
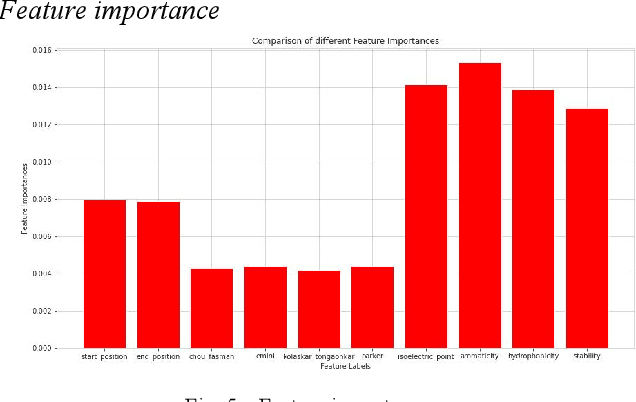
The accurate prediction of B-cell epitopes is critical for guiding vaccine development against infectious diseases, including SARS and COVID-19. This study explores the use of a deep neural network (DNN) model to predict B-cell epitopes for SARS-CoVandSARS-CoV-2,leveraging a dataset that incorporates essential protein and peptide features. Traditional sequence-based methods often struggle with large, complex datasets, but deep learning offers promising improvements in predictive accuracy. Our model employs regularization techniques, such as dropout and early stopping, to enhance generalization, while also analyzing key features, including isoelectric point and aromaticity, that influence epitope recognition. Results indicate an overall accuracy of 82% in predicting COVID-19 negative and positive cases, with room for improvement in detecting positive samples. This research demonstrates the applicability of deep learning in epitope mapping, suggesting that such approaches can enhance the speed and precision of vaccine design for emerging pathogens. Future work could incorporate structural data and diverse viral strains to further refine prediction capabilities.

Mode Connectivity in Auction Design

Optimal auction design is a fundamental problem in algorithmic game theory. This problem is notoriously difficult already in very simple settings. Recent work in differentiable economics showed that neural networks can efficiently learn known optimal auction mechanisms and discover interesting new ones. In an attempt to theoretically justify their empirical success, we focus on one of the first such networks, RochetNet, and a generalized version for affine maximizer auctions. We prove that they satisfy mode connectivity, i.e., locally optimal solutions are connected by a simple, piecewise linear path such that every solution on the path is almost as good as one of the two local optima. Mode connectivity has been recently investigated as an intriguing empirical and theoretically justifiable property of neural networks used for prediction problems. Our results give the first such analysis in the context of differentiable economics, where neural networks are used directly for solving non-convex optimization problems.