Yang Junwei
Is Perfect Filtering Enough Leading to Perfect Phase Correction for dMRI data?
Jun 13, 2021
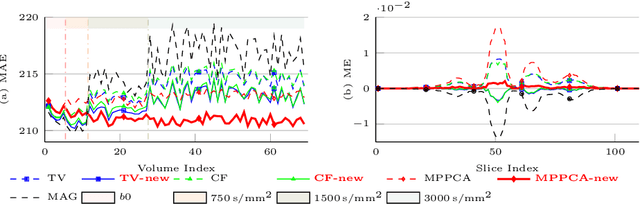


Abstract:Being complex-valued and low in signal-to-noise ratios, magnitude-based diffusion MRI is confounded by the noise-floor that falsely elevates signal magnitude and incurs bias to the commonly used diffusion indices, such as fractional anisotropy (FA). To avoid noise-floor, most existing phase correction methods explore improving filters to estimate the noise-free background phase. In this work, after diving into the phase correction procedures, we argue that even a perfect filter is insufficient for phase correction because the correction procedures are incapable of distinguishing sign-symbols of noise, resulting in artifacts (\textit{i.e.}, arbitrary signal loss). With this insight, we generalize the definition of noise-floor to a complex polar coordinate system and propose a calibration procedure that could conveniently distinguish noise sign symbols. The calibration procedure is conceptually simple and easy to implement without relying on any external technique while keeping distinctly effective.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge