Sudhan Majhi
A New Method of Constructing Hadamard Matrices, Circulant Hadamard Matrices, CZCS, GCS, CCC, and CZCSS
Oct 14, 2025Abstract:A Hadamard matrix $H$ is a square matrix of order $n$ with entries $\pm 1$, such that $HH^\top=nI_{n}$, where $I_n$ is an identity matrix of order $n$. A circulant Hadamard matrix $H$ is a Hadamard matrix that has rows of entries in cyclic order. There exist only $8$ circulant Hadamard matrices of order 4, and here, we provide a novel construction of all such $8$ circulant Hadamard matrices using a linear operator and generalized Boolean function (GBF). The constructed circulant Hadamard matrices are used recursively to construct a binary cross Z-complementary set (CZCS) of all lengths with an even phase, a binary Golay complementary set (GCS) of all lengths, and Hadamard matrices of order $2^{n+2}$, where $n\geq1$. The construction of a binary CZCS covering all lengths was not available before. We also propose an alternative, lower-complexity construction of binary GCSs of all lengths and Hadamard matrices of order $2^{a+1}10^b26^c$ using circulant matrices, where $ a,b,c \geq 0$. The proposed binary GCS covers all lengths with a flexible flock size. The constructions of GCS are further extended to form binary complete complementary code (CCC) of the parameter $(2N,2N,2N)-CCC$ where $N=2^a10^b26^c, a,b,c \geq 0$. The constructed binary CCC provides a flexible flock size. The construction of CZCS is further extended to form a binary optimal cross-Z complementary sequence set (CZCSS) of the parameter $(2^{n+2}, 2^{n+2}, 2^{n+2}, 2^{n+1})-CZCSS$, where $n\geq1$. Finally, we provide a relation between Hadamard matrices and GCS, which enables the study of the Hadamard conjecture in a new direction. We also provided a few properties of circulant matrices over aperiodic cross-correlation (ACCF) and aperiodic auto-correlation (AACF), which are used to prove the theorems. All proposed constructions are novel, and their parameters are compared with the existing state-of-the-art.
Asymptotically Optimal Quasi-Complementary Code Sets from Multivariate Functions
Apr 28, 2022
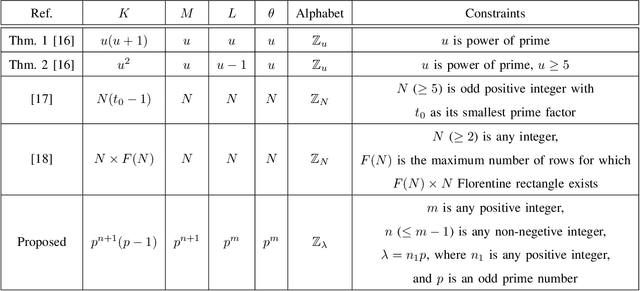
Abstract:Owing to the more significant set size properties as compared to the set of complete complementary codes (CCCs), quasi-complementary code sets (QCCSs) are more convenient to support a large number of users in multicarrier code-division multiple-access (MC-CDMA) system over CCCs. Besides set size, it is also desirable to have a low maximum aperiodic correlation magnitude and small alphabet size. This paper aims to construct asymptotically optimal and near-optimal aperiodic QCCSs having a small alphabet size and low maximum correlation magnitude. Using multivariate functions and its associated graph, we propose a family of QCCSs consisting of multiple sets of CCCs and determine the parameters of the proposed QCCSs. Unlike the existing constructions of aperiodic QCCSs, the proposed construction can maintain a small alphabet size irrespective of the increasing sequence length and large set size.
Pseudo-Boolean Functions for Optimal Z-Complementary Code Sets with Flexible Lengths
Apr 20, 2021
Abstract:This paper aims to construct optimal Z-complementary code set (ZCCS) with non-power-of-two (NPT) lengths to enable interference-free multicarrier code-division multiple access (MC-CDMA) systems. The existing ZCCSs with NPT lengths, which are constructed from generalized Boolean functions (GBFs), are sub-optimal only with respect to the set size upper bound. For the first time in the literature, we advocate the use of pseudo-Boolean functions (PBFs) (each of which transforms a number of binary variables to a real number as a natural generalization of GBF) for direct constructions of optimal ZCCSs with NPT lengths.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge