Permutation-equivariant quantum convolutional neural networks

The Symmetric group $S_{n}$ manifests itself in large classes of quantum systems as the invariance of certain characteristics of a quantum state with respect to permuting the qubits. The subgroups of $S_{n}$ arise, among many other contexts, to describe label symmetry of classical images with respect to spatial transformations, e.g. reflection or rotation. Equipped with the formalism of geometric quantum machine learning, in this work we propose the architectures of equivariant quantum convolutional neural networks (EQCNNs) adherent to $S_{n}$ and its subgroups. We demonstrate that a careful choice of pixel-to-qubit embedding order can facilitate easy construction of EQCNNs for small subgroups of $S_{n}$. Our novel EQCNN architecture corresponding to the full permutation group $S_{n}$ is built by applying all possible QCNNs with equal probability, which can also be conceptualized as a dropout strategy in quantum neural networks. For subgroups of $S_{n}$, our numerical results using MNIST datasets show better classification accuracy than non-equivariant QCNNs. The $S_{n}$-equivariant QCNN architecture shows significantly improved training and test performance than non-equivariant QCNN for classification of connected and non-connected graphs. When trained with sufficiently large number of data, the $S_{n}$-equivariant QCNN shows better average performance compared to $S_{n}$-equivariant QNN . These results contribute towards building powerful quantum machine learning architectures in permutation-symmetric systems.

The role of data embedding in equivariant quantum convolutional neural networks

Geometric deep learning refers to the scenario in which the symmetries of a dataset are used to constrain the parameter space of a neural network and thus, improve their trainability and generalization. Recently this idea has been incorporated into the field of quantum machine learning, which has given rise to equivariant quantum neural networks (EQNNs). In this work, we investigate the role of classical-to-quantum embedding on the performance of equivariant quantum convolutional neural networks (EQCNNs) for the classification of images. We discuss the connection between the data embedding method and the resulting representation of a symmetry group and analyze how changing representation affects the expressibility of an EQCNN. We numerically compare the classification accuracy of EQCNNs with three different basis-permuted amplitude embeddings to the one obtained from a non-equivariant quantum convolutional neural network (QCNN). Our results show that all the EQCNNs achieve higher classification accuracy than the non-equivariant QCNN for small numbers of training iterations, while for large iterations this improvement crucially depends on the used embedding. It is expected that the results of this work can be useful to the community for a better understanding of the importance of data embedding choice in the context of geometric quantum machine learning.

Experimental quantum pattern recognition in IBMQ and diamond NVs

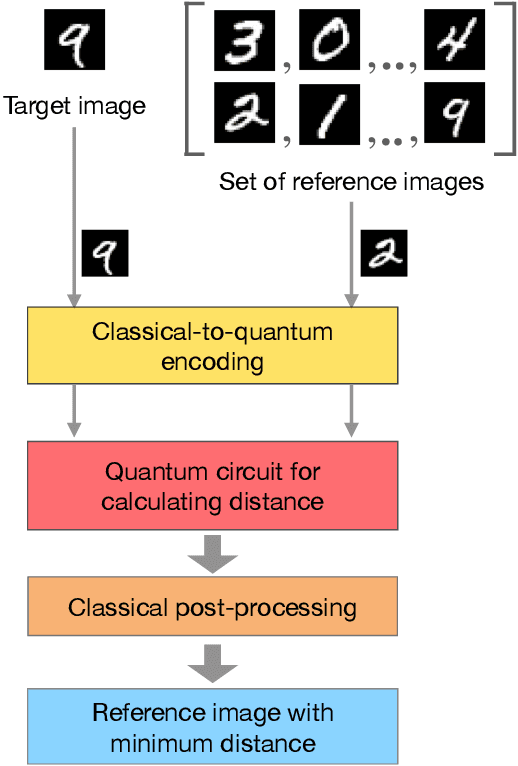
One of the most promising applications of quantum computing is the processing of graphical data like images. Here, we investigate the possibility of realizing a quantum pattern recognition protocol based on swap test, and use the IBMQ noisy intermediate-scale quantum (NISQ) devices to verify the idea. We find that with a two-qubit protocol, swap test can efficiently detect the similarity between two patterns with good fidelity, though for three or more qubits the noise in the real devices becomes detrimental. To mitigate this noise effect, we resort to destructive swap test, which shows an improved performance for three-qubit states. Due to limited cloud access to larger IBMQ processors, we take a segment-wise approach to apply the destructive swap test on higher dimensional images. In this case, we define an average overlap measure which shows faithfulness to distinguish between two very different or very similar patterns when simulated on real IBMQ processors. As test images, we use binary images with simple patterns, greyscale MNIST numbers and MNIST fashion images, as well as binary images of human blood vessel obtained from magnetic resonance imaging (MRI). We also present an experimental set up for applying destructive swap test using the nitrogen vacancy centre (NVs) in diamond. Our experimental data show high fidelity for single qubit states. Lastly, we propose a protocol inspired from quantum associative memory, which works in an analogous way to supervised learning for performing quantum pattern recognition using destructive swap test.

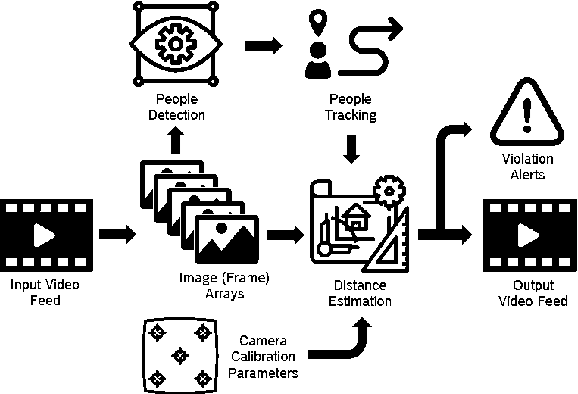
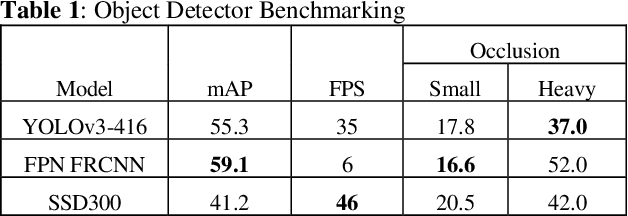
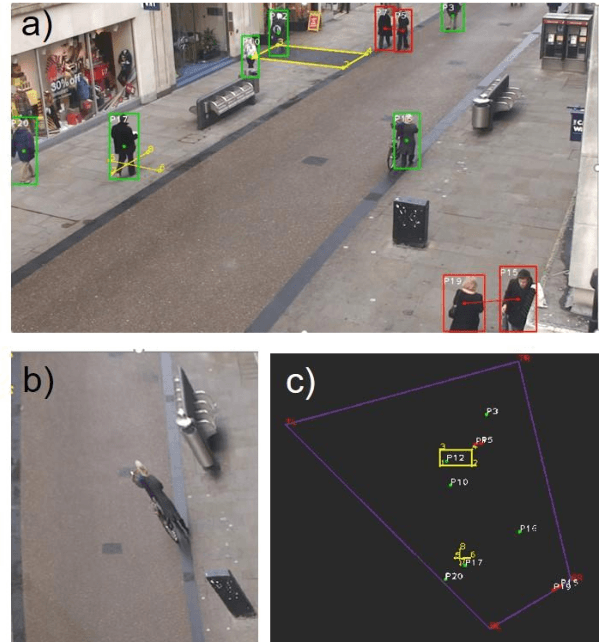
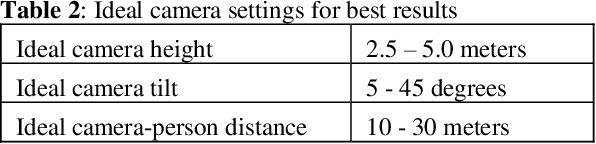
Computer Vision-based Social Distancing Surveillance Solution with Optional Automated Camera Calibration for Large Scale Deployment

Social distancing has been suggested as one of the most effective measures to break the chain of viral transmission in the current COVID-19 pandemic. We herein describe a computer vision-based AI-assisted solution to aid compliance with social distancing norms. The solution consists of modules to detect and track people and to identify distance violations. It provides the flexibility to choose between a tool-based mode or an automated mode of camera calibration, making the latter suitable for large-scale deployments. In this paper, we discuss different metrics to assess the risk associated with social distancing violations and how we can differentiate between transient or persistent violations. Our proposed solution performs satisfactorily under different test scenarios, processes video feed at real-time speed as well as addresses data privacy regulations by blurring faces of detected people, making it ideal for deployments.