Coarse-graining conformational dynamics with multi-dimensional generalized Langevin equation: how, when, and why

A data-driven ab initio generalized Langevin equation (AIGLE) approach is developed to learn and simulate high-dimensional, heterogeneous, coarse-grained conformational dynamics. Constrained by the fluctuation-dissipation theorem, the approach can build coarse-grained models in dynamical consistency with all-atom molecular dynamics. We also propose practical criteria for AIGLE to enforce long-term dynamical consistency. Case studies of a toy polymer, with 20 coarse-grained sites, and the alanine dipeptide, with two dihedral angles, elucidate why one should adopt AIGLE or its Markovian limit for modeling coarse-grained conformational dynamics in practice.

Coarse-grained spectral projection (CGSP): A scalable and parallelizable deep learning-based approach to quantum unitary dynamics

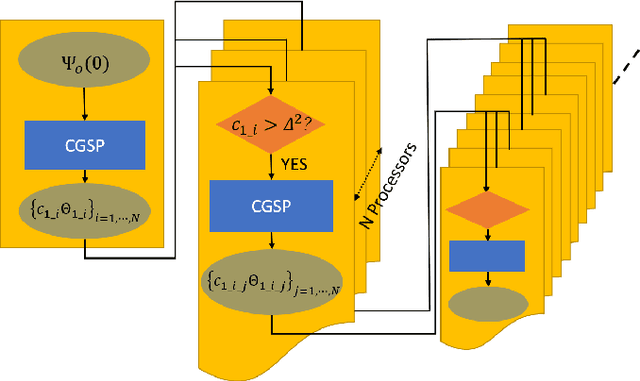
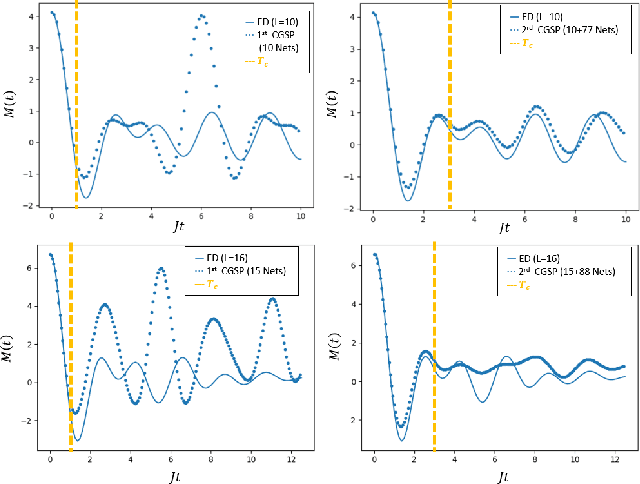
We propose the coarse-grained spectral projection method (CGSP), a deep learning approach for tackling quantum unitary dynamic problems with an emphasis on quench dynamics. We show CGSP can extract spectral components of many-body quantum states systematically with highly entangled neural network quantum ansatz. CGSP exploits fully the linear unitary nature of the quantum dynamics, and is potentially superior to other quantum Monte Carlo methods for ergodic dynamics. Practical aspects such as naturally parallelized implementations on modern deep learning infrastructures are also discussed. Preliminary numerical experiments are carried out to guide future development of CGSP.