PC-DeepNet: A GNSS Positioning Error Minimization Framework Using Permutation-Invariant Deep Neural Network

Global navigation satellite systems (GNSS) face significant challenges in urban and sub-urban areas due to non-line-of-sight (NLOS) propagation, multipath effects, and low received power levels, resulting in highly non-linear and non-Gaussian measurement error distributions. In light of this, conventional model-based positioning approaches, which rely on Gaussian error approximations, struggle to achieve precise localization under these conditions. To overcome these challenges, we put forth a novel learning-based framework, PC-DeepNet, that employs a permutation-invariant (PI) deep neural network (DNN) to estimate position corrections (PC). This approach is designed to ensure robustness against changes in the number and/or order of visible satellite measurements, a common issue in GNSS systems, while leveraging NLOS and multipath indicators as features to enhance positioning accuracy in challenging urban and sub-urban environments. To validate the performance of the proposed framework, we compare the positioning error with state-of-the-art model-based and learning-based positioning methods using two publicly available datasets. The results confirm that proposed PC-DeepNet achieves superior accuracy than existing model-based and learning-based methods while exhibiting lower computational complexity compared to previous learning-based approaches.

On Comparison between Evolutionary Programming Network-based Learning and Novel Evolution Strategy Algorithm-based Learning

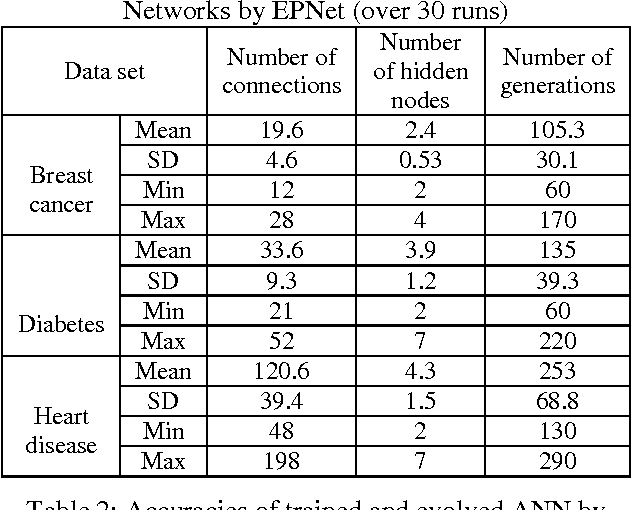
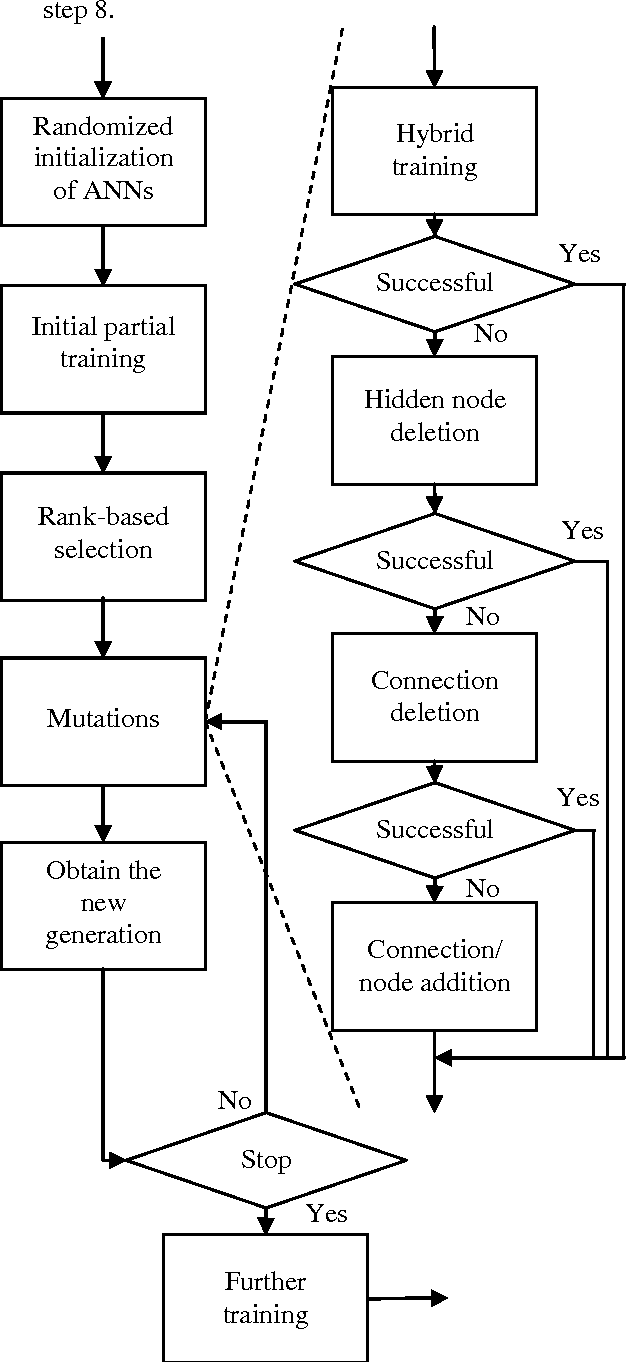
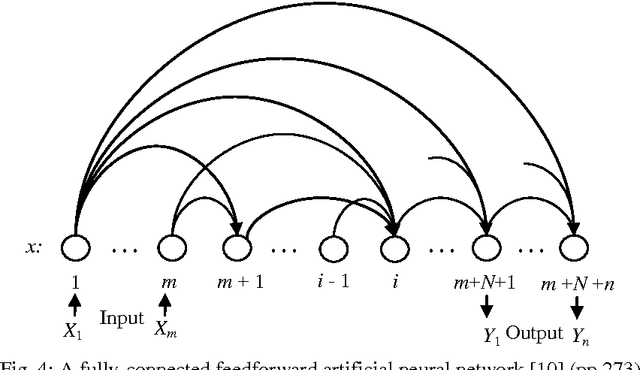
This paper presents two different evolutionary systems - Evolutionary Programming Network (EPNet) and Novel Evolutions Strategy (NES) Algorithm. EPNet does both training and architecture evolution simultaneously, whereas NES does a fixed network and only trains the network. Five mutation operators proposed in EPNet to reflect the emphasis on evolving ANNs behaviors. Close behavioral links between parents and their offspring are maintained by various mutations, such as partial training and node splitting. On the other hand, NES uses two new genetic operators - subpopulation-based max-mean arithmetical crossover and time-variant mutation. The above-mentioned two algorithms have been tested on a number of benchmark problems, such as the medical diagnosis problems (breast cancer, diabetes, and heart disease). The results and the comparison between them are also presented in this paper.