Elliot Padgett
Sampling Limits for Electron Tomography with Sparsity-exploiting Reconstructions
Apr 04, 2019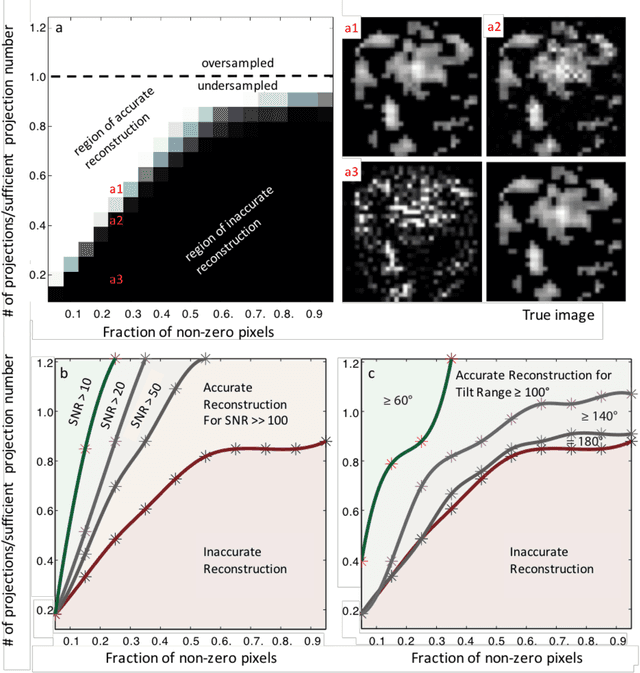
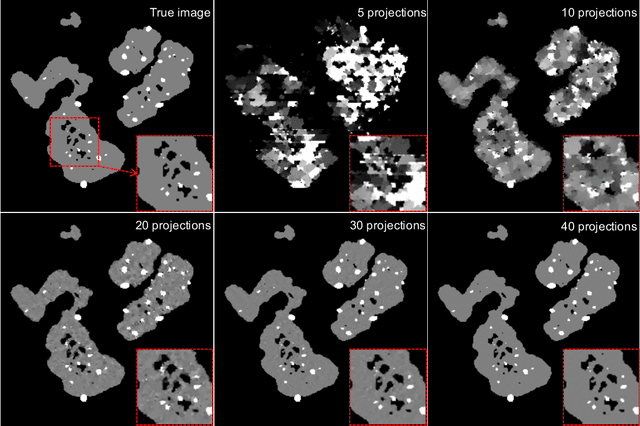
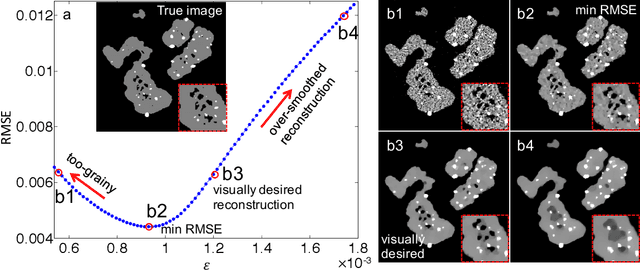
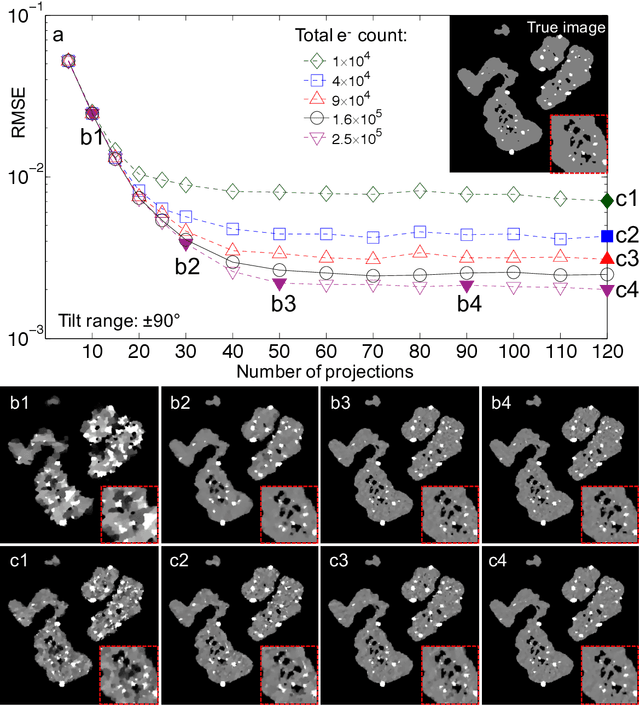
Abstract:Electron tomography (ET) has become a standard technique for 3D characterization of materials at the nano-scale. Traditional reconstruction algorithms such as weighted back projection suffer from disruptive artifacts with insufficient projections. Popularized by compressed sensing, sparsity-exploiting algorithms have been applied to experimental ET data and show promise for improving reconstruction quality or reducing the total beam dose applied to a specimen. Nevertheless, theoretical bounds for these methods have been less explored in the context of ET applications. Here, we perform numerical simulations to investigate performance of l_1-norm and total-variation (TV) minimization under various imaging conditions. From 36,100 different simulated structures, our results show specimens with more complex structures generally require more projections for exact reconstruction. However, once sufficient data is acquired, dividing the beam dose over more projections provides no improvements - analogous to the traditional dose-fraction theorem. Moreover, a limited tilt range of +-75 or less can result in distorting artifacts in sparsity-exploiting reconstructions. The influence of optimization parameters on reconstructions is also discussed.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge