Deep Convolutional Neural Network for Low Projection SPECT Imaging Reconstruction

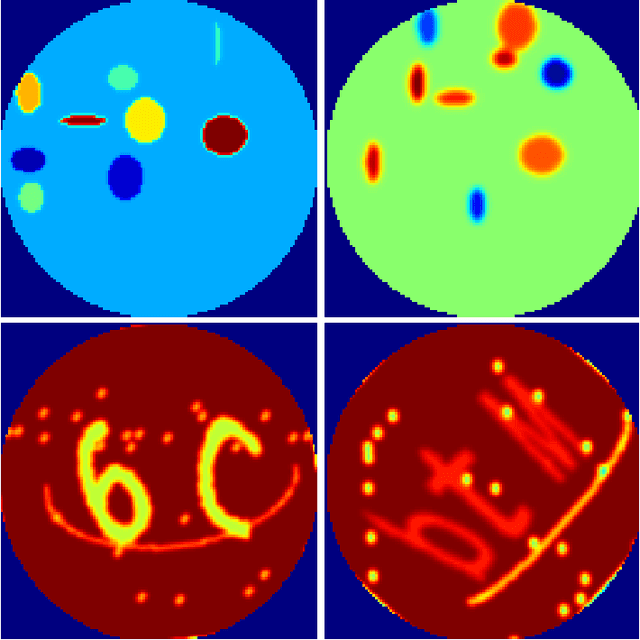
In this paper, we present a novel method for tomographic image reconstruction in SPECT imaging with a low number of projections. Deep convolutional neural networks (CNN) are employed in the new reconstruction method. Projection data from software phantoms were used to train the CNN network. For evaluation of the efficacy of the proposed method, software phantoms and hardware phantoms based on the FOV SPECT system were used. The resulting tomographic images are compared to those produced by the "Maximum Likelihood Expectation Maximisation" (MLEM).

SPECT Angle Interpolation Based on Deep Learning Methodologies

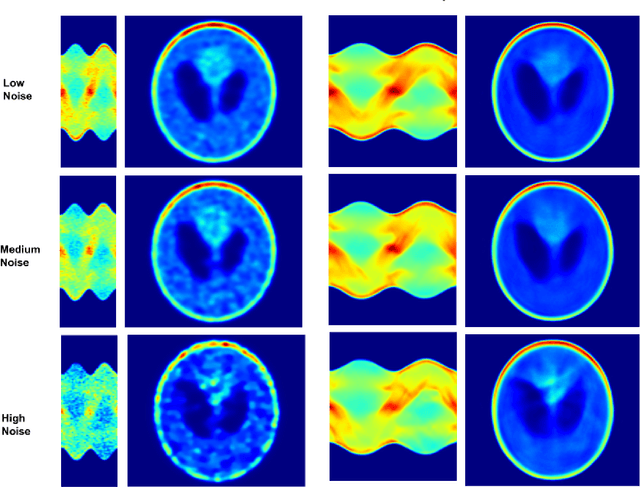
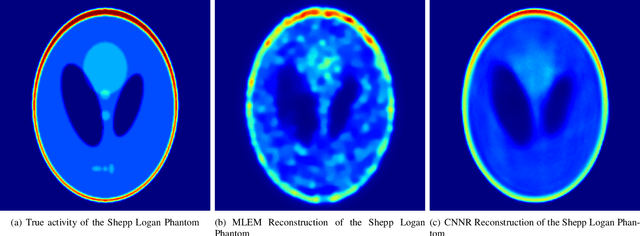
A novel method for SPECT angle interpolation based on deep learning methodologies is presented. Projection data from software phantoms were used to train the proposed model. For evaluation of the efficacy of the method, phantoms based on Shepp Logan, with various noise levels added were used, and the resulting interpolated sinograms are reconstructed using Ordered Subset Expectation Maximization (OSEM) and compared to the reconstructions of the original sinograms. The proposed method can quadruple the projections, and denoise the original sinogram, in the same process. As the results show, the proposed model significantly improves the reconstruction accuracy. Finally, to demonstrate the efficacy and capability of the proposed method results from real-world DAT-SPECT sinograms are presented.

SPECT Imaging Reconstruction Method Based on Deep Convolutional Neural Network

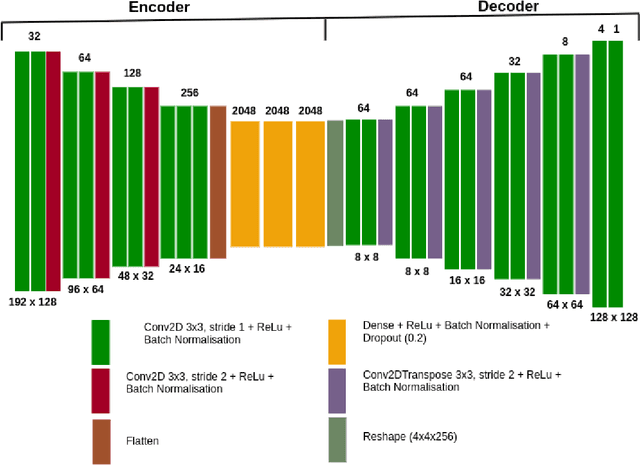
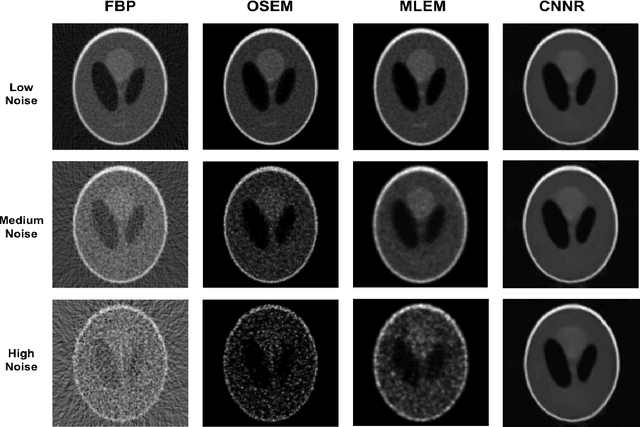
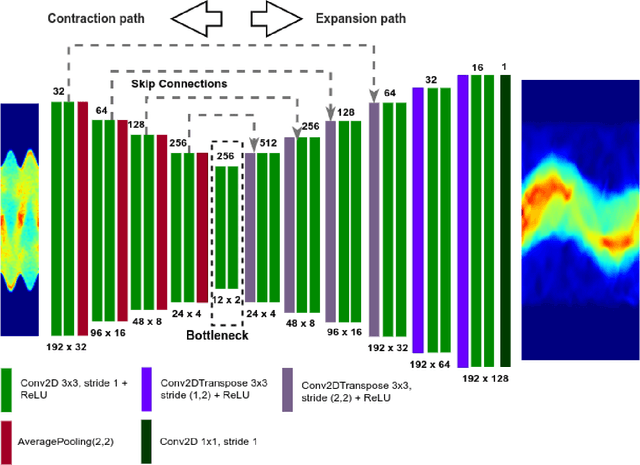
In this paper, we explore a novel method for tomographic image reconstruction in the field of SPECT imaging. Deep Learning methodologies and more specifically deep convolutional neural networks (CNN) are employed in the new reconstruction method, which is referred to as "CNN Reconstruction - CNNR". For training of the CNNR Projection data from software phantoms were used. For evaluation of the efficacy of the CNNR method, both software and hardware phantoms were used. The resulting tomographic images are compared to those produced by filtered back projection (FBP) [1], the "Maximum Likelihood Expectation Maximization" (MLEM) [1] and ordered subset expectation maximization (OSEM) [2].