The Complete Extensions do not form a Complete Semilattice
Paper and Code
Oct 26, 2017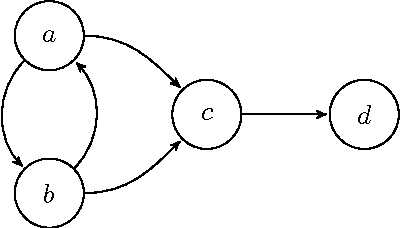
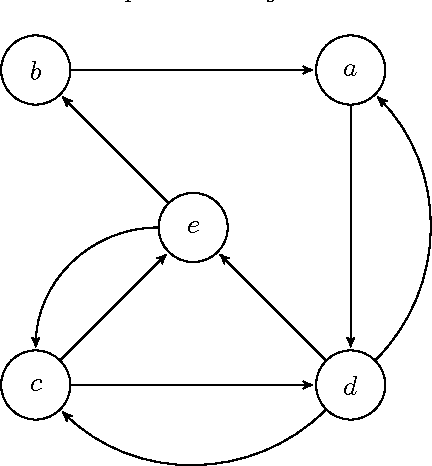
In his seminal paper that inaugurated abstract argumentation, Dung proved that the set of complete extensions forms a complete semilattice with respect to set inclusion. In this note we demonstrate that this proof is incorrect with counterexamples. We then trace the error in the proof and explain why it arose. We then examine the implications for the grounded extension. [Reason for withdrawal continued] Page 4, Example 2 is not a counterexample to Dung 1995 Theorem 25(3). It was believed to be a counter-example because the author misunderstood ``glb'' to be set-theoretic intersection. But in this case, ``glb'' is defined to be other than set-theoretic intersection such that Theorem 25(3) is true. The author was motivated to fully understand the lattice-theoretic claims of Dung 1995 in writing this note and was not aware that this issue is probably folklore; the author bears full responsibility for this error.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge