Stress-Based Navigation for Microscopic Robots in Viscous Fluids
Paper and Code
Nov 23, 2018
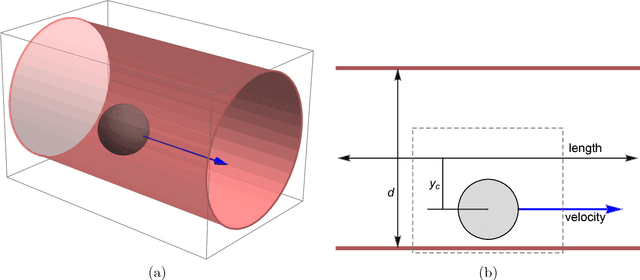

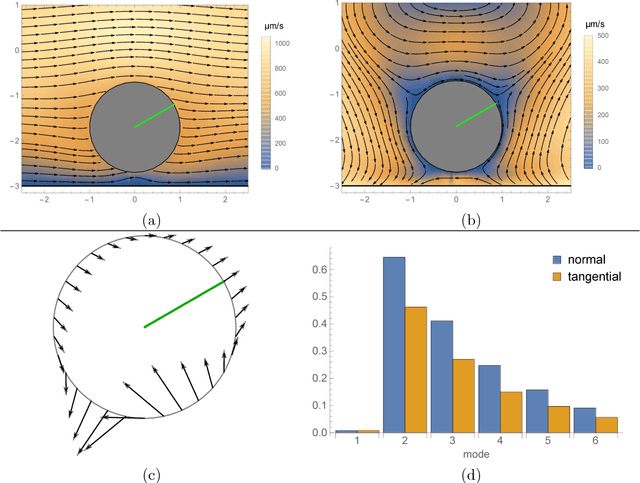
Objects moving in fluids experience patterns of stress on their surfaces determined by their motion and the geometry of nearby boundaries. Fish and underwater robots can use these patterns for navigation. This paper extends this stress-based navigation to microscopic robots in tiny vessels, where robots can exploit the physics of fluids at low Reynolds number. This applies, for instance, in vessels with sizes and flow speeds comparable to those of capillaries in biological tissues. We describe how a robot can use simple computations to estimate its motion, orientation and distance to nearby vessel walls from fluid-induced stresses on its surface. Numerically evaluating these estimates for a variety of vessel sizes and robot positions shows they are most accurate when robots are close to vessel walls.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge