SAR Image Despeckling Algorithms using Stochastic Distances and Nonlocal Means
Paper and Code
Aug 20, 2013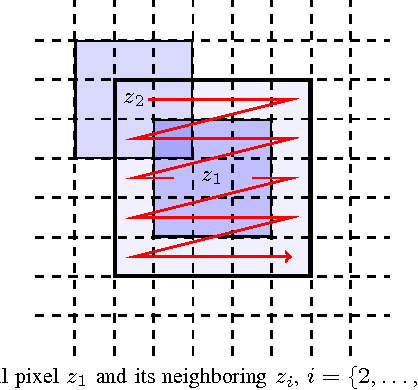
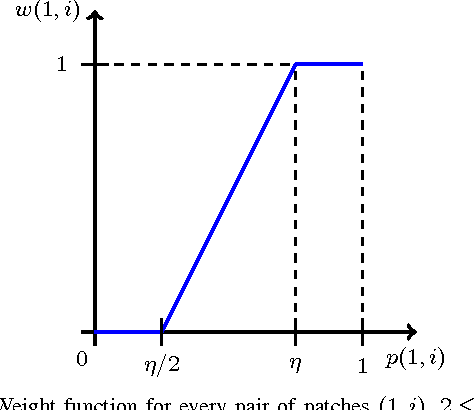
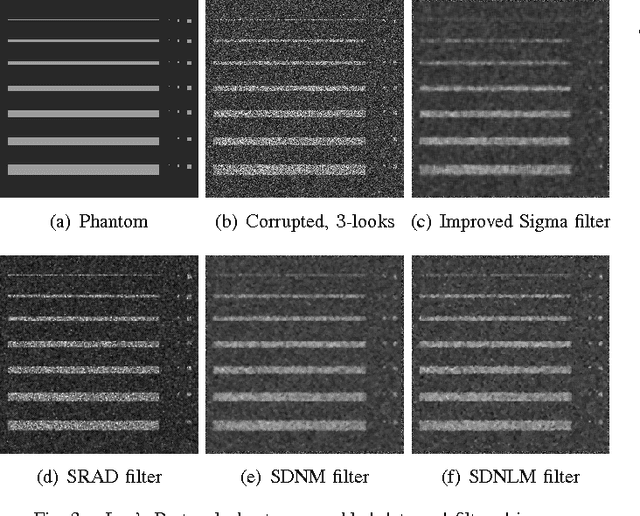
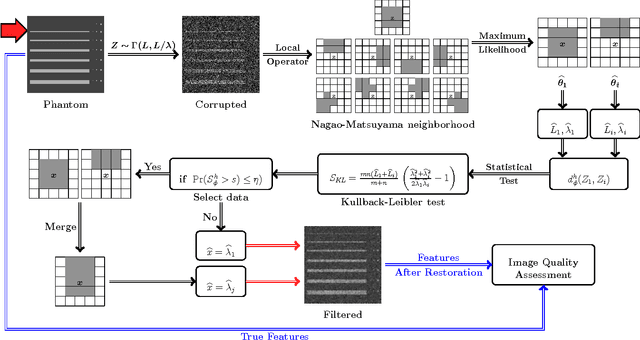
This paper presents two approaches for filter design based on stochastic distances for intensity speckle reduction. A window is defined around each pixel, overlapping samples are compared and only those which pass a goodness-of-fit test are used to compute the filtered value. The tests stem from stochastic divergences within the Information Theory framework. The technique is applied to intensity Synthetic Aperture Radar (SAR) data with homogeneous regions using the Gamma model. The first approach uses a Nagao-Matsuyama-type procedure for setting the overlapping samples, and the second uses the nonlocal method. The proposals are compared with the Improved Sigma filter and with anisotropic diffusion for speckled data (SRAD) using a protocol based on Monte Carlo simulation. Among the criteria used to quantify the quality of filters, we employ the equivalent number of looks, and line and edge preservation. Moreover, we also assessed the filters by the Universal Image Quality Index and by the Pearson correlation between edges. Applications to real images are also discussed. The proposed methods show good results.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge