Optimal but Low-Complexity Optimization Method for Nonsquare Differential Massive MIMO
Paper and Code
May 26, 2023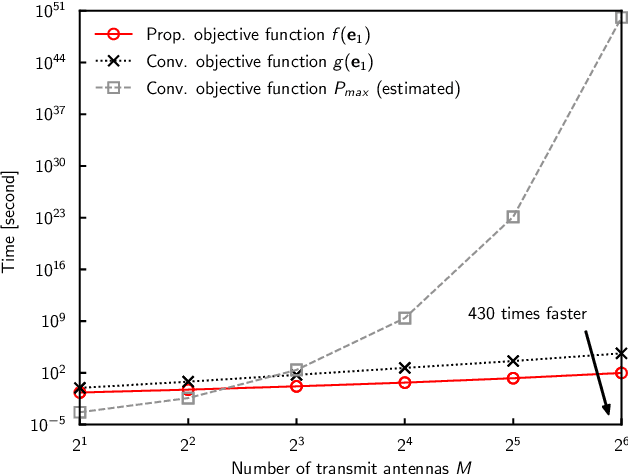
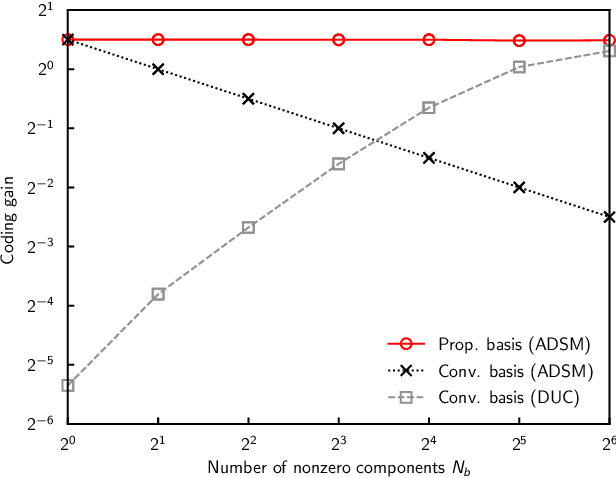
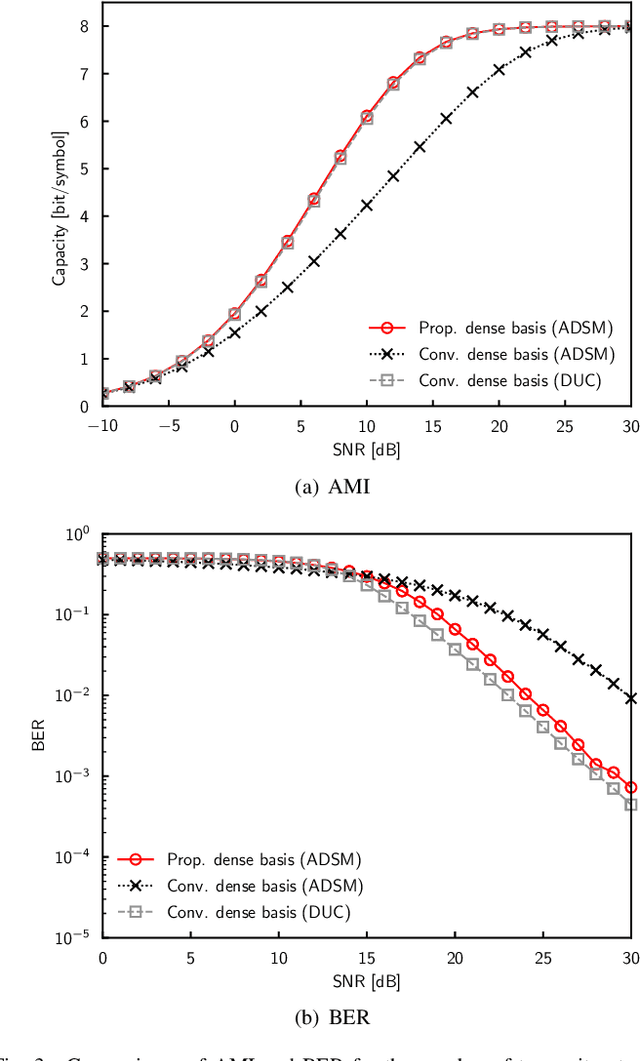
In this paper, we propose an optimal but low-complexity optimization method for nonsquare differential massive MIMO. While a discrete nonlinear optimization is required for the conventional nonsquare differential coding, we newly modify it to perform a low-complexity continuous linear optimization. This novel method exhibits immediate convergence as compared to the conventional method. Additionally, the proposed differential coding can be regarded as a differential counterpart of the coherent generalized spatial modulation. Our numerical comparisons demonstrate that the proposed method achieves the best coding gain for any number of transmit antennas, although the optimization cost is nearly negligible.
* IEEE 94th Vehicular Technology Conference (VTC2021-Fall) * 5 pages, 4 figures
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge