On the Realization of Impulse Invariant Bilinear Volterra Kernels
Paper and Code
Jul 12, 2021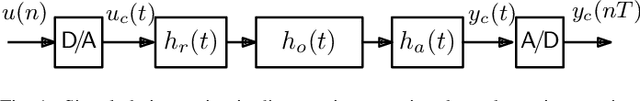
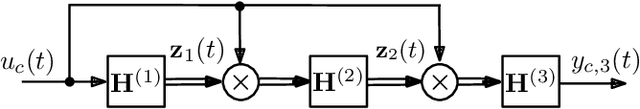
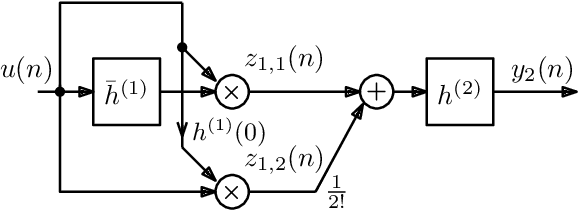

As previously shown, the direct extension of the impulse invariance principle to Volterra kernels has to be modified in order to provide a condition for the exact modeling of mixed-signal chains. At first sight this would seem to seriously complicate the otherwise simple discrete-time realization of separable kernels (among which bilinear kernels are of particular importance). We show here, however, that this not the case. By defining a cascade operator, the structure of a generalized impulse invariance can be unveiled, leading to a realization without an inordinate increase in computational complexity.
* 5 pages, 4 figures
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge