Modeling dispersive silver in the electrodynamic lattice-Boltzmann method using complex-conjugate pole-residue pairs
Paper and Code
Sep 30, 2022

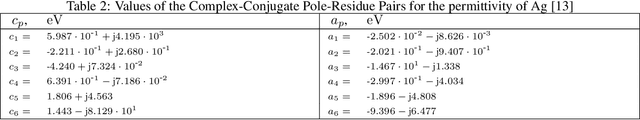

The polarization density of a broadband electrodynamic lattice-Boltzmann method (ELBM) is generalized to represent frequency-dispersion of materials interacting with electromagnetic waves. The frequency-dependent refractive index and extinction coefficient are modeled using complex-conjugate pole-residue pairs in an auxiliary-differential-equation (ADE). Electric and magnetic fields are evaluated on a single lattice, ensuring a stable numerical solution up to the Nyquist limit. The electric and magnetic fields from the ELBM are compared with the electric and magnetic fields from the finite-difference-time-domain (FDTD) method. Accurate transmittance of a 100 nm silver slab is extracted from the transmitted power spectrum of a broadband Dirac-delta wave-function for photon energies ranging from 0.125-5 eV. Given this capability, the ELBM with an ADE is an accurate and computationally efficient method for modeling broadband frequency-dispersion of materials.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge