Jeopardy: An Invertible Functional Programming Language

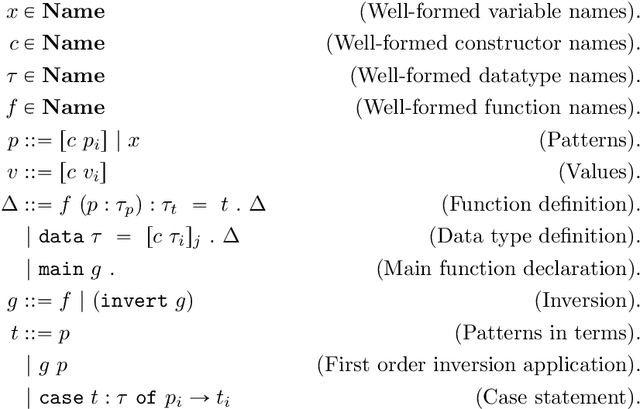
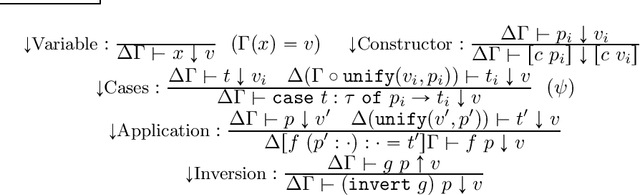
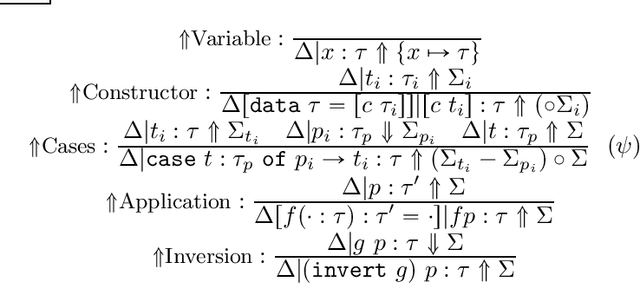
An algorithm describes a sequence of steps that transform a problem into its solution. Furthermore, when the inverted sequence is well-defined, we say that the algorithm is invertible. While invertible algorithms can be described in general-purpose languages, no guarantees are generally made by such languages as regards invertibility, so ensuring invertibility requires additional (and often non-trivial) proof. On the other hand, while reversible programming languages guarantee that their programs are invertible by restricting the permissible operations to those which are locally invertible, writing programs in the reversible style can be cumbersome, and may differ significantly from conventional implementations even when the implemented algorithm is, in fact, invertible. In this paper we introduce Jeopardy, a functional programming language that guarantees program invertibility without imposing local reversibility. In particular, Jeopardy allows the limited use of uninvertible -- and even nondeterministic! -- operations, provided that they are used in a way that can be statically determined to be invertible. However, guaranteeing invertibility is not obvious. Thus, we furthermore outline three approaches that can give a partial static guarantee.