Continuum-Armed Bandits: A Function Space Perspective
Paper and Code
Oct 26, 2020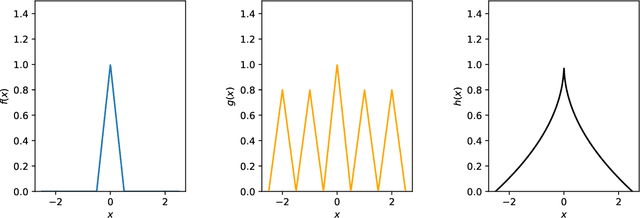
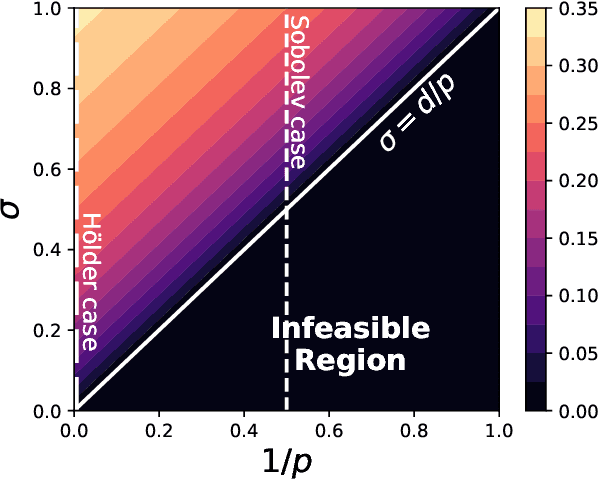
Continuum-armed bandits (a.k.a., black-box or $0^{th}$-order optimization) involves optimizing an unknown objective function given an oracle that evaluates the function at a query point, with the goal of using as few query points as possible. In the most well-studied case, the objective function is assumed to be Lipschitz continuous and minimax rates of simple and cumulative regrets are known in both noiseless and noisy settings. This paper studies continuum-armed bandits under more general smoothness conditions, namely Besov smoothness conditions, on the objective function. In both noiseless and noisy conditions, we derive minimax rates under simple and cumulative regrets. Our results show that minimax rates over objective functions in a Besov space are identical to minimax rates over objective functions in the smallest H\"older space into which the Besov space embeds.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge