Approximate Integer Solution Counts over Linear Arithmetic Constraints
Paper and Code
Dec 14, 2023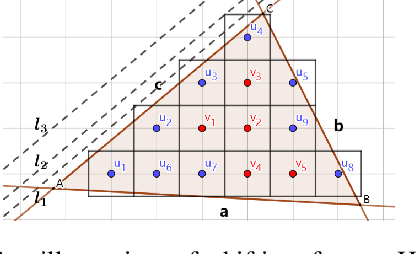
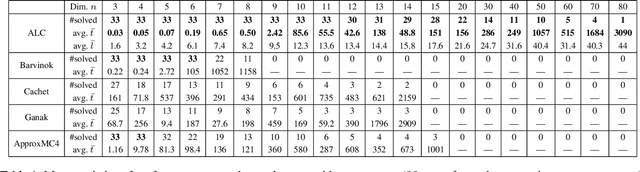
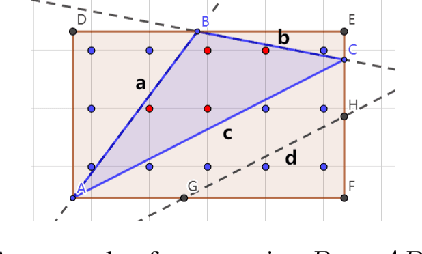
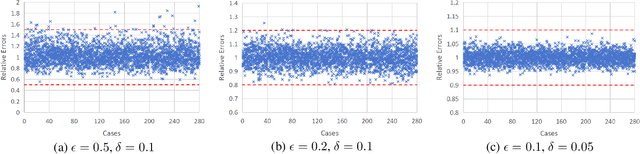
Counting integer solutions of linear constraints has found interesting applications in various fields. It is equivalent to the problem of counting lattice points inside a polytope. However, state-of-the-art algorithms for this problem become too slow for even a modest number of variables. In this paper, we propose a new framework to approximate the lattice counts inside a polytope with a new random-walk sampling method. The counts computed by our approach has been proved approximately bounded by a $(\epsilon, \delta)$-bound. Experiments on extensive benchmarks show that our algorithm could solve polytopes with dozens of dimensions, which significantly outperforms state-of-the-art counters.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge