Antenna Optimization Using a New Evolutionary Algorithm Based on Tukey-Lambda Probability Distribution
Paper and Code
Jun 06, 2020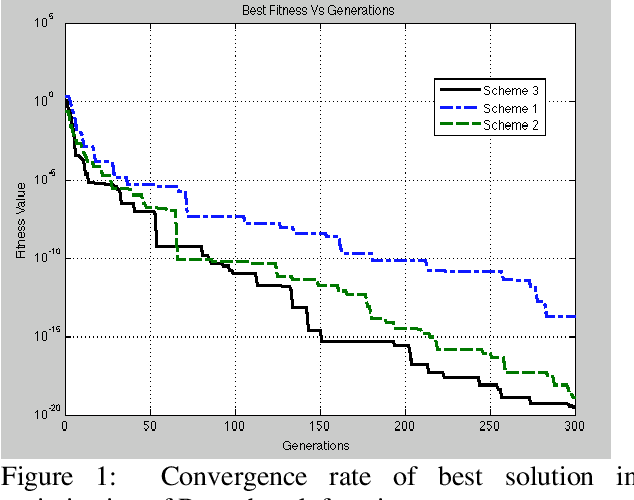
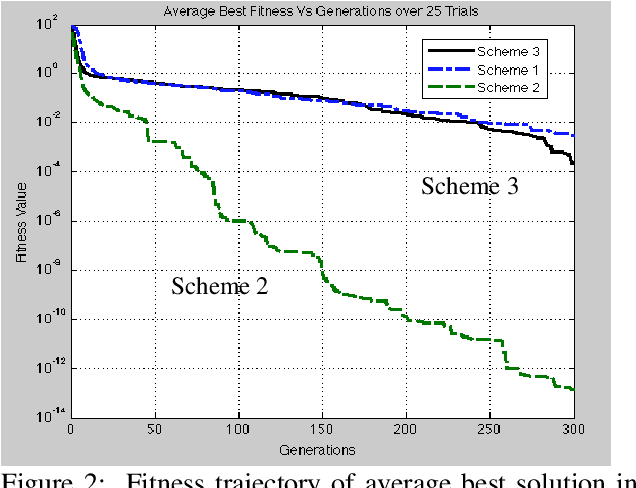
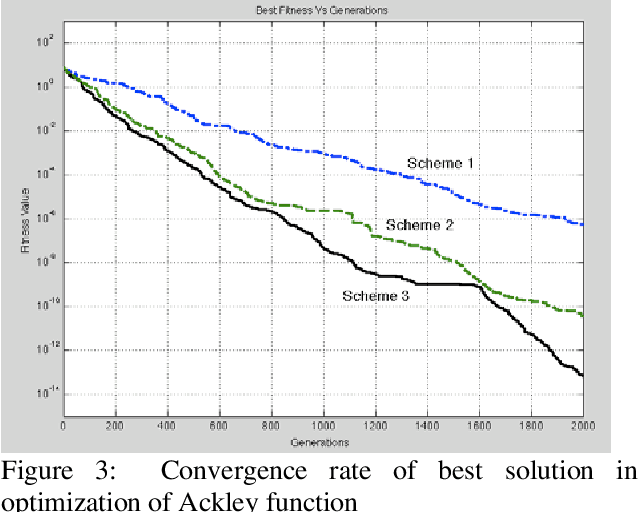
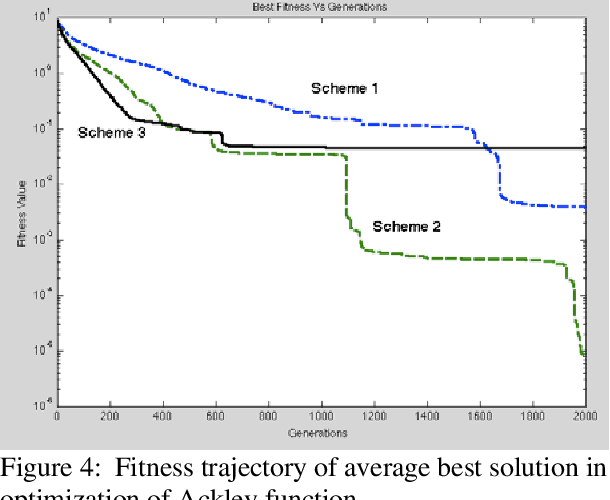
In this paper, we introduce a new evolutionary optimization algorithm based on Tukey's symmetric lambda distribution. Tukey distribution is defined by 3 parameters, the shape parameter, the scale parameter, and the location parameter or average value. Various other distributions can be approximated by changing the shape parameter, and as a result can encompass a large class of probability distributions. In addition, Because of these attributes, an Evolutionary Programming (EP) algorithm with Tukey mutation operator may perform well in a large class of optimization problems. Various schemes in implementation of EP with Tukey distribution are discussed, and the resulting algorithms are applied to selected test functions and antenna design problems.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge